Eyes Wide
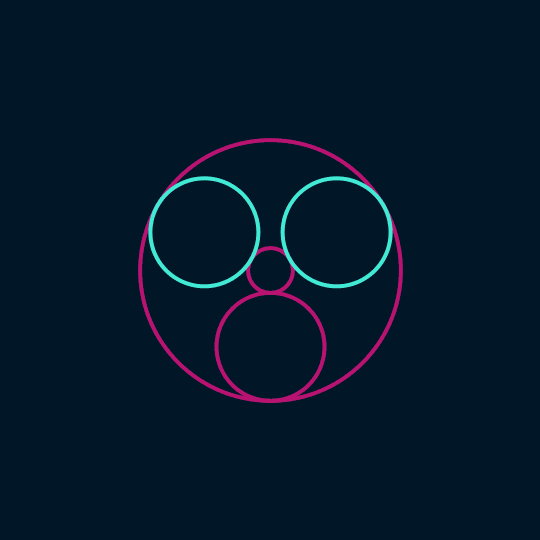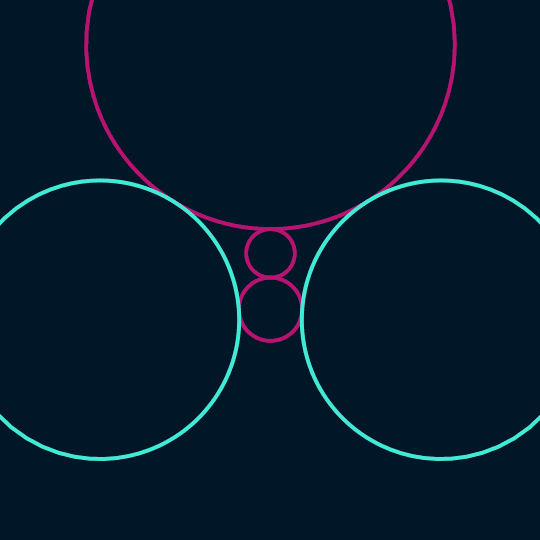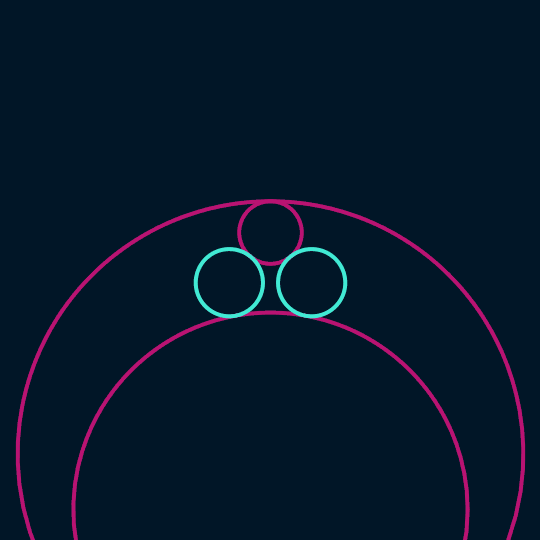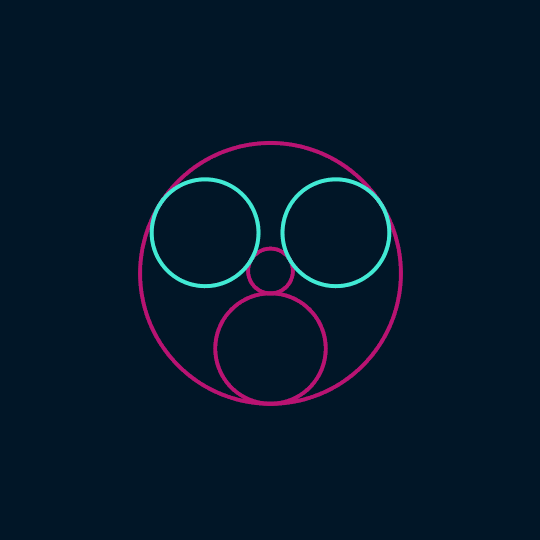
Very much in the same spirit as Pack It In, this shows the stereographic image of the optimal packing of the sphere by five circles. The circles are centered at the (normalized) vertices of the triangular bipyramid.
The coloration is misleading, in that the five circles are partitioned into a group of three (the three centered on points $120^\circ$ apart on the equator) and a group of two (the two centered on the north and south poles), but the colors don't match up with those groupings. The two blue circles are two of the three circles centered on the equator; the third is the one circle that doesn't touch those two blue circles.
Anyway, here's the code:
Stereo[p_] := Most[p]/(1 - Last[p]);
DynamicModule[{p, b, t = ?/4.,
verts =
RotationTransform[?/6, {0, 0, 1}][Normalize[N[#]]] & /@ PolyhedronData[{"Dipyramid", 3}, "VertexCoordinates"],
cols = RGBColor /@ {"#B91372", "#41EAD4", "#011627"}},
Manipulate[
p = verts.RotationMatrix[-?, {1, 0, 0}];
b = Orthogonalize[NullSpace[{#}]] & /@ p;
Graphics[
{FaceForm[None],
Table[
{EdgeForm[
Directive[Thickness[.0075], cols[[Mod[Ceiling[i/3], 2, 1]]]]],
Polygon[Table[Stereo[Cos[t] p[[i]] + Sin[t] (Cos[s] b[[i, 1]] + Sin[s] b[[i, 2]])], {s, 0, 2 ?, 2 ?/200}]]},
{i, 1, Length[verts]}]},
PlotRange -> 5, Background -> cols[[-1]], ImageSize -> 540],
{?, 0, 2 ?}]
]