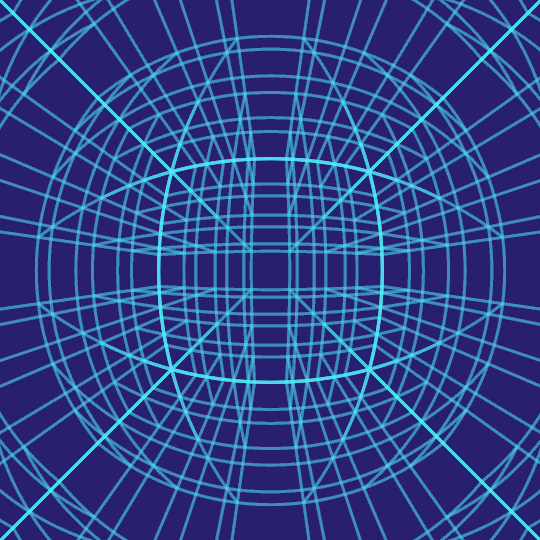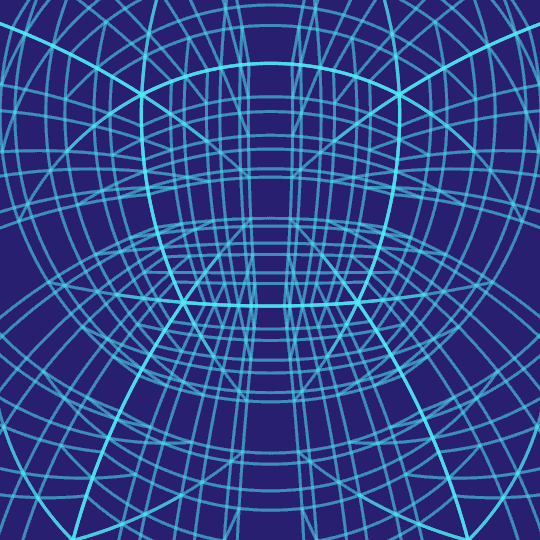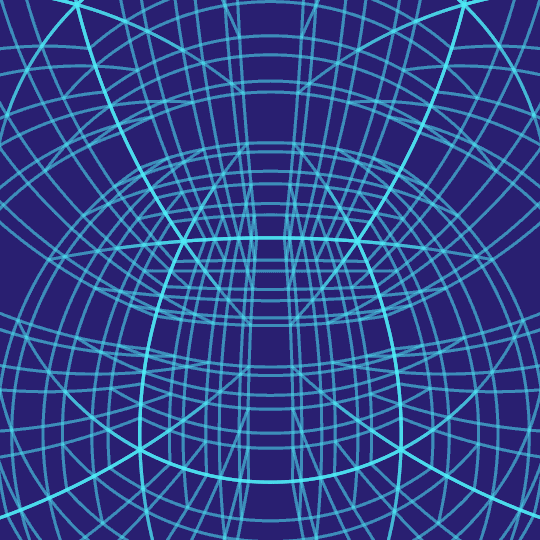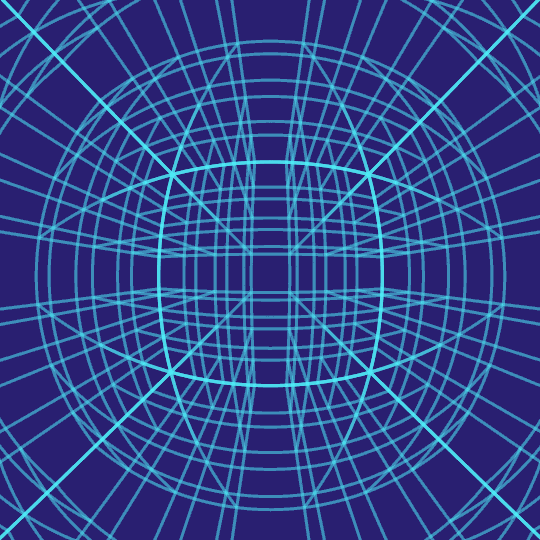
Microcosm
This is conceptually very simple: take a $7 \times 7$ grid of unit cubes in space, normalize to the unit sphere, stereographically project to the plane, then apply a rotation to the original grid of cubes. Here's the code:
Stereo[p_] := 1/(1 - p[[-1]]) p[[;; 2]];
With[{n = 3, cols = RGBColor /@ {"#4EEAF6", "#291F71"}},
Manipulate[
Graphics[
{Opacity[.5], CapForm[None], cols[[1]], Thickness[.006],
Line[
Flatten[
Transpose[
Table[
Stereo[Normalize[#]] & /@
{{t,
y Cos[?] - z Sin[?],
z Cos[?] + y Sin[?]},
{y, t Cos[?] - z Sin[?],
z Cos[?] + t Sin[?]},
{y, z Cos[?] - t Sin[?],
t Cos[?] + z Sin[?]}},
{z, -n - 1/2, n + 1/2}, {y, -n - 1/2, n + 1/2}, {t, -n - 1/2.,
n + 1/2, 1/20}],
{2, 3, 4, 1, 5}],
2]
]
},
PlotRange -> 1, Axes -> False, ImageSize -> 540, Background -> cols[[-1]]],
{?, 0, ?/2}]
]