Abstract
This notebook presents tables for addition and subtraction that have a better use of the place value system. The method is already used in Holland for addition in levels but this notebook extends for addition in differences and subtraction in levels and differences. The method is only intended for an intermediate stage in teaching before addition and subtraction are mentally fully automated. For example 99 + 21 can be added per digit position. To keep digits in the range [0, 9] we remove underflow or overflow. We work from right to left, since the numbers come from India and Arabia. Then we get 99 + 21 -> {9, 9} + {2, 1} = {11, 10} = {11, 10} + {1, -10} = {12, 0} = {0, 12, 0} + {1, -10, 0} = {1, 2, 0} -> 120. Compared to existing methods: (1) This method does not change the original sum. (2) The workflow is into a single direction. (3) Allowing positions to have values outside the [0 , 9] range focuses attention upon the place value. (4) There is a unity of approach to both addition and subtraction. The method fits within the US Common Core when we tell kids that a step with {1, -10} represents the subtraction 1 * 10 - 10 * 1 = 0. It would be more fundamental to adapt the curriculum for negative numbers though. Routines to create such tables for addition and subtraction are available in a package. Obviously pupils in elementary school must master the method by hand, but the package allows for examples and checking.
NB. The notebook and package have been updated on May 5. The html text below might be the original text of April 2. The following link has the same name of April but contains the updated notebook and package:
https://www.wolframcloud.com/objects/thomas-cool/MathEd/2018-04-02-AdditionTable-and-SubtractionTable.nb
The notebook and package in the attachment have also been updated. See also https://zenodo.org/record/1241405
Introduction
There is a more efficient way for tables of addition and subtraction, see Colignatus (2014), that takes better advantage of the place value system (decimal system). This would suit the Common Core (2018) objectives.
The methods are now available in a package. Obviously pupils in elementary school must master the method by hand, but the package allows for examples and checking.
Discussion
To teach the place value system, it is advisable to add or subtract per position.
- This does not change the original sum.
- The workflow is into a single direction, not jumping around to the beginning and back.
- Allowing positions to have values outside the [0 , 9] range focuses attention upon the place value.
- There is a unity of approach to both addition and subtraction.
I have not seen this method elsewhere, so if you start using this, please refer to this present source, so that others can find the proper documentation.
See also Colignatus (2014), also for the use of enclosing circles, or colours, or brackets for overflow or underflow positions, with numbers like 9 [11]7 = 1017 = [11][ -9 ]7.
{9, 11, 7} - {11, -9, 7}
{-2,20,0}
Wikipedia is a portal and no source, and compare: https://en.wikipedia.org/wiki/Subtraction#Subtraction_by_hand
With steps of differences
That these tables use differences can be seen from the diagonal form.
AdditionTable[99, 21]
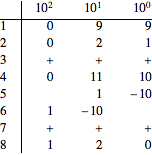
AdditionTable[999, 777, 123]
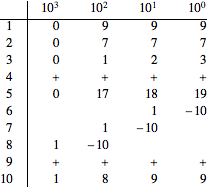
SubtractionTable[491, 753]
With steps in levels
That these tables use levels can be seen from the repeat of the place values.
AdditionTable[99, 21, Differences -> False]
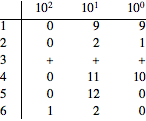
AdditionTable[999, 777, 123, Differences -> False]
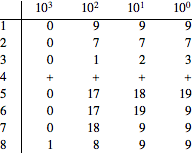
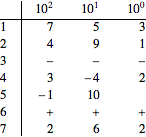
SubtractionTable[753, 491, Differences -> False]

Calculation strategy
Pupils must also develop some calculation strategies:
- Addition allows that more numbers are added at the same time, and the order doesn't matter.
- Simple subtraction has only two numbers, the largest minus the smallest. If one really wants to subtract a large number from a smaller one, multiply the outcome by -1. If there are more numbers to subtract, first add all numbers that must be subtracted.
- Involved substraction uses more than two numbers. In this case, there can be a negative outcome. One must be aware that a number like -123 will be displayed as {-1, -2, -3}.
Subtraction in other order or with more numbers
Here one must make a rough judgement whether the outcome will be negative or positive.
SubtractionTable[{11, 99}]

SubtractionTable[11, 99, 78]
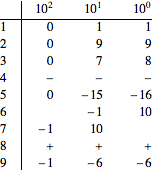
SubtractionTable[999, 11, 99, 78]
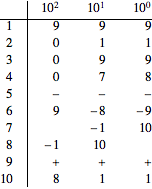
The routines
?Cool`MathEd`AdditionTable`*

?AdditionTable

?SubtractionTable
Conclusions
It is obviously advantageous when tables of addition and subtraction use the place value system in a better manner.
The place value system by nature supports this way of working. It is rather amazing that this method has not been developed before. Likely it has been a mental stumbling block that one doesn't allow for overflow or underflow per digit position, at least in writing, since one must allow for it in the mind, namely to record the overflow or underflow.
Literature
Thomas Colignatus is the scientific name of Thomas Cool, econometrician and teacher of mathematics, Scheveningen, Holland.
Colignatus, Th. (2014), Taking a loss, https://boycottholland.wordpress.com/2014/08/30/taking-a-loss/
Colignatus, Th. (2009, 2015a), Elegance with Substance,
(1) website: http://thomascool.eu/Papers/Math/Index.html,
(2) PDF on Zenodo: https://zenodo.org/record/291974,
(3) Publisher for a print: https://www.mijnbestseller.nl/shop/index.php/catalog/product/view/id/115148/s/elegance-with-substance-78023-www-mijnbestseller-nl/
Colignatus, Th. (2015b), A child wants nice and no mean numbers,
(1) website: http://thomascool.eu/Papers/NiceNumbers/Index.html
(2) PDF on Zenodo: https://zenodo.org/record/291979
(3) Publisher for a print: https://www.mijnbestseller.nl/shop/index.php/catalog/product/view/id/118082/s/a-child-wants-nice-and-no-mean-numbers-79074-www-mijnbestseller-nl/
Colignatus, Th. (2018), Arithmetic with H = -1 and proper mixed numbers and fractions, https://www.wolframcloud.com/objects/thomas-cool/MathEd/2018-02-04-Arithmetic-with-H.nb
Common Core Standards (2018), Number & Operations in Base Ten, http://www.corestandards.org/Math/Content/NBT/ with also Understand place value
 Attachments:
Attachments: