Recut

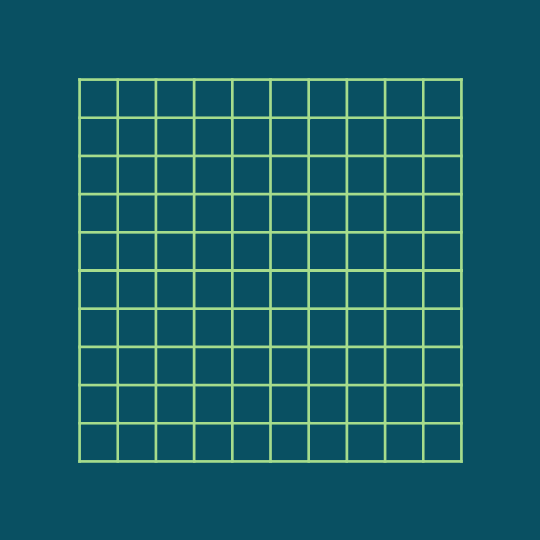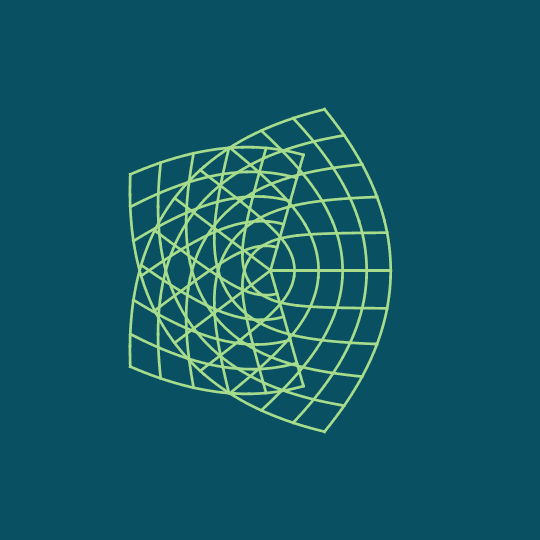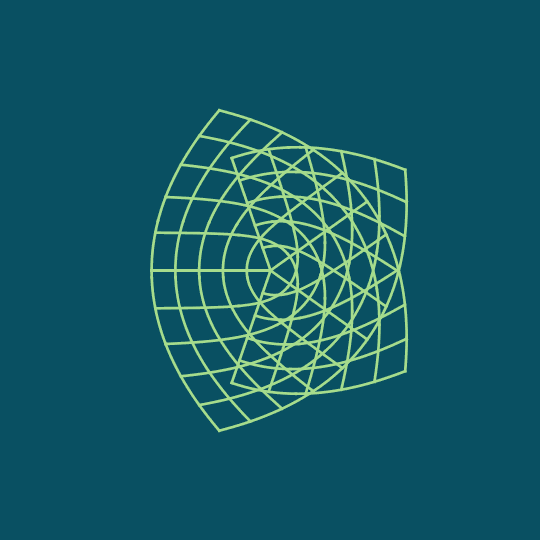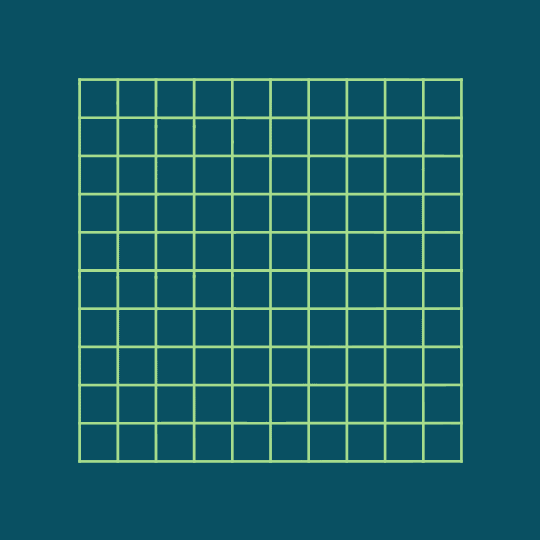
This one is pretty simple: take the unit square, cut a slit halfway into it on one side, fold into cones of smaller and smaller angle, and project to the plane. Inspired by the MathWorld page on cone nets.

Here's the code for half the animation (to get the other half, flip across the y-axis and reverse the time direction):

Manipulate[Module[{xstep, ystep, cols, curvestyle},
  xstep = 1/5;
  ystep = 1/5;
  cols = RGBColor /@ {"#A6DC8C", "#095062"};
  curvestyle = Directive[Thickness[.005], cols[[1]]];
  Show[ParametricPlot[
    Table[{r n Cos[\[Theta]/n], 
       r n Sin[\[Theta]/n]} /. {\[Theta] -> ArcTan[x, y], 
       r -> Sqrt[x^2 + y^2]}, {x, -1, 1, xstep}], {y, 0, 1}, 
    PlotRange -> Sqrt[2], Axes -> False, ImageSize -> 540, 
    PlotStyle -> curvestyle, Background -> cols[[2]]], 
   ParametricPlot[
    Table[{r n Cos[\[Theta]/n], 
       r n Sin[\[Theta]/n]} /. {\[Theta] -> ArcTan[x, y], 
       r -> Sqrt[x^2 + y^2]}, {x, -1, 1, xstep}], {y, -1, -.0001}, 
    PlotStyle -> curvestyle], 
   ParametricPlot[
    Table[{r n Cos[\[Theta]/n], 
       r n Sin[\[Theta]/n]} /. {\[Theta] -> ArcTan[x, y], 
       r -> Sqrt[x^2 + y^2]}, {y, 
      Append[Range[-1, 1, ystep], -.0001]}], {x, -1, 1}, 
    PlotStyle -> curvestyle]]
  ], {n, 1/2, 1}]