Good Vibrations
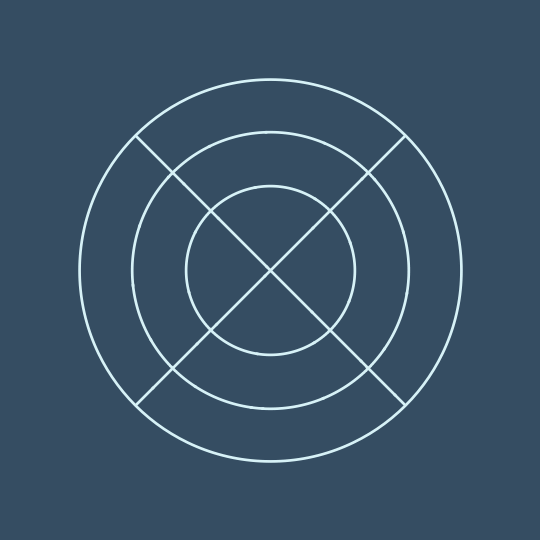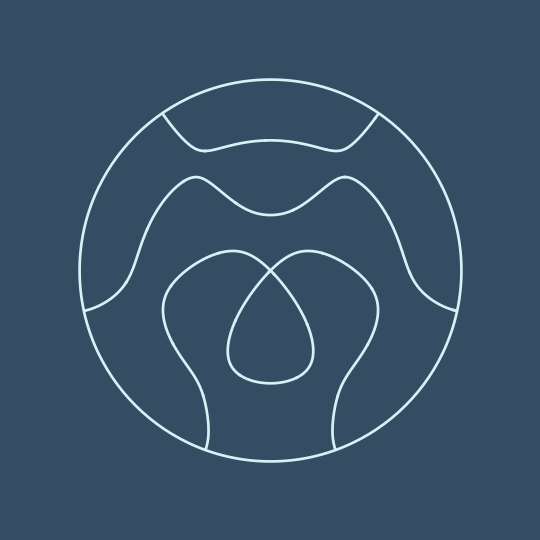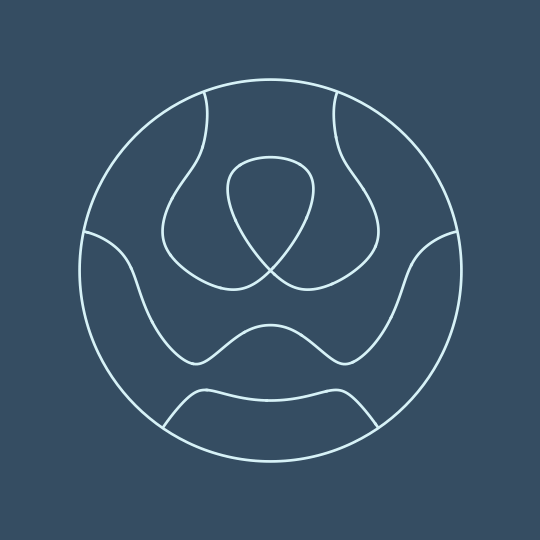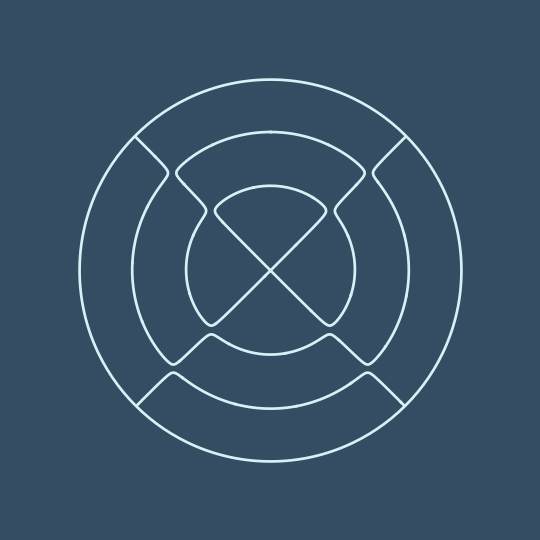
In the same spirit as The Band Plays On, here are Chladni patterns (which is to say: the nodal lines) for a family of vibration modes on the disk interpolating between the $(2,3)$ mode and the $(3,2)$ mode. ContourPlot is pretty slow for this, so rather than an unresponsive Manipulate, here's the code for generating the GIF:
BesselZeros = N@Table[BesselJZero[m, n], {m, 0, 5}, {n, 1, 5}];
?[m_, n_] := BesselZeros[[m + 1, n]];
u[m_, n_, r_, ?_, t_, c_, A_, B_, C_, D_]
:= (A Cos[c ?[m, n] t] + B Sin[c ?[m, n] t])
BesselJ[m, ?[m, n] r] (C Cos[m ?] + D Sin[m ?]);
diskChladni = With[
{m = 1,
n = 2,
c = 1,
cols = RGBColor /@ {"#D7F2F7", "#354D62"}},
ParallelTable[
Show[
Graphics[{Thickness[.005], cols[[1]], Circle[]}],
ContourPlot[
Cos[t] u[2, 3, Norm[{-y, x}], ArcTan[-y, x], 0, c, 1/2, 0, 1/2, 0]
+ Sin[t] u[3, 2, Norm[{-y, x}], ArcTan[-y, x], 0, c, 1/2, 0, 1/2, 0] == 0,
{x, -1, 1}, {y, -1, 1}, PlotPoints -> 100,
ContourStyle -> Directive[Thickness[.005], cols[[1]]],
RegionFunction -> Function[{x, y, z}, x^2 + y^2 < .99]],
Background -> cols[[-1]], PlotRange -> Sqrt[2],
ImageSize -> 540],
{t, 0., ? - #, #}] &[?/200]
];
Export[NotebookDirectory[] <> "diskChladni.gif", diskChladni, "DisplayDurations" -> {3/100}]