Go Deeper

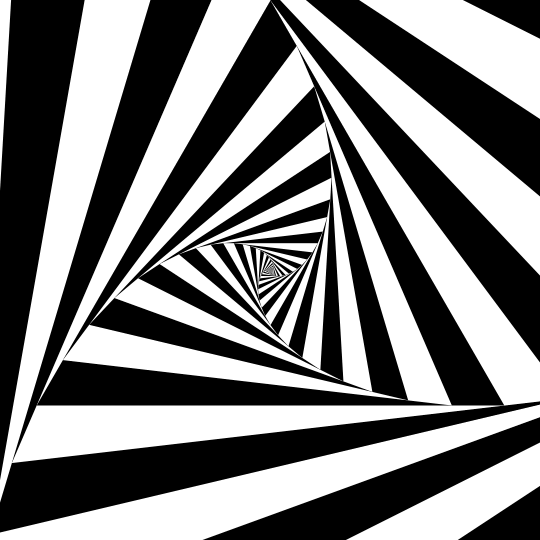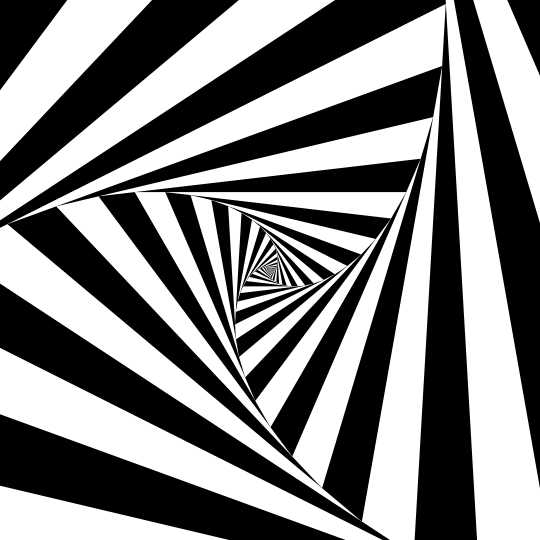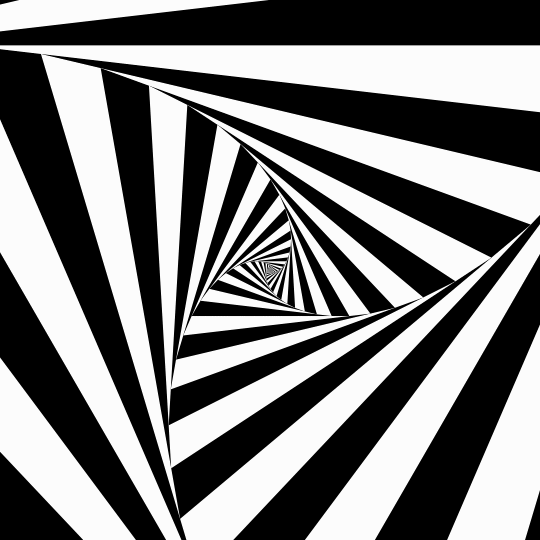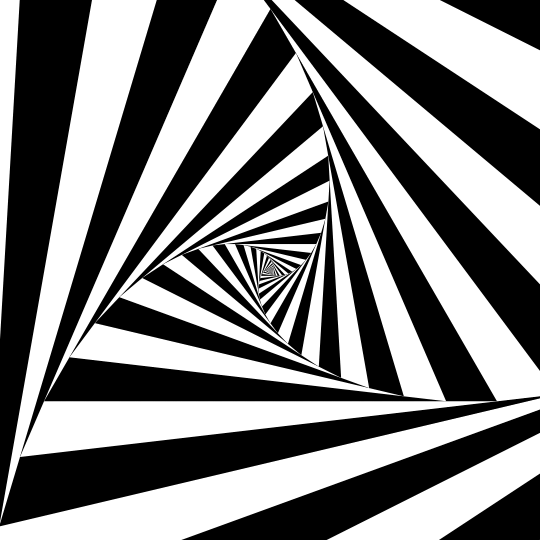
Following up on Step Out, this is a classic whirl with the twist of making it into an infinite zoom, which I've always wanted to do but never had a good idea for.

If you look at the code, you'll see a weird weight factor of $w=0.22448400191341356$, meaning that at each level of recursion the vertices of the triangle at that level lie at a fraction $0.22448400191341356/2 = 0.11224200095670678$ of the way along an edge of the triangle at the previous level. This weight factor ensures that the whole whirl is self-similar (without having to rotate the orientation) with a scaling factor of $\approx 24.442985761484984$.

I found this weight in a pretty simple-minded way, by just turning the weight into a Manipulate parameter, playing around until it was approximately self-similar, then finding a more precise answer using NSolve.

Anyway, I think that's probably the only super-mysterious part of the code:

WeightedMidpoints[pts_, w_] := Mean[({w #, (2 - w) RotateLeft[#]} &)[pts]]

DynamicModule[{n = 3, w = 0.22448400191341356, depth = 150, cols = {Black, White}, a, pts, t},
 pts = CirclePoints[n];
 a = Nest[WeightedMidpoints[#, w] &, CirclePoints[3], 18][[3, 2]];
 Manipulate[
  Graphics[{
    Table[{
        FaceForm[If[OddQ[i], cols[[1]], cols[[2]]]], Polygon[#[[i]]]},
       {i, 1, Length[#]}] &@NestList[WeightedMidpoints[#, w] &, pts, depth]},
   PlotRange -> E^(s Log[a]), ImageSize -> 540],
  {s, 1, 2}]
 ]