Canis

I've been playing around lately with stereographic projection and Möbius transformations of the circle. This is neither stereographic projection nor a Möbius transformation, but it came out of the same collection of ideas.

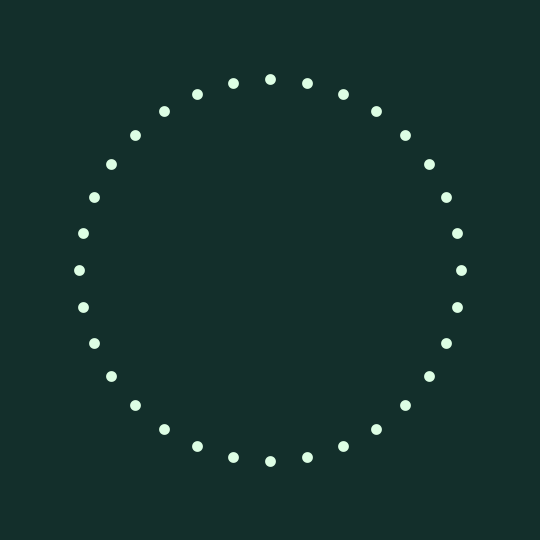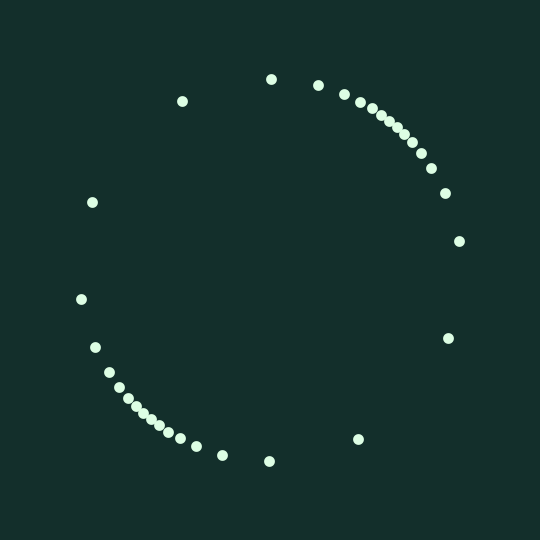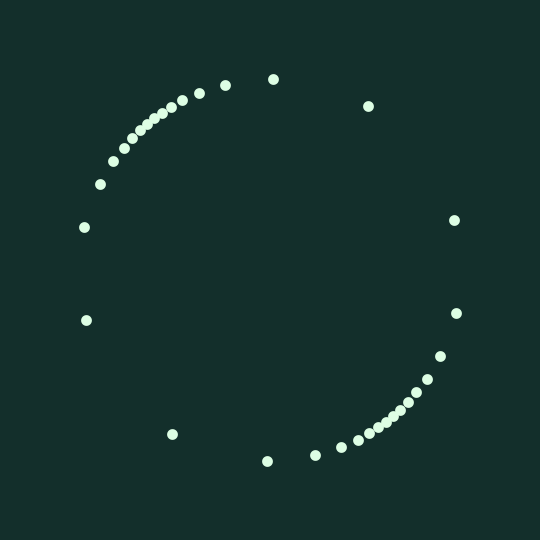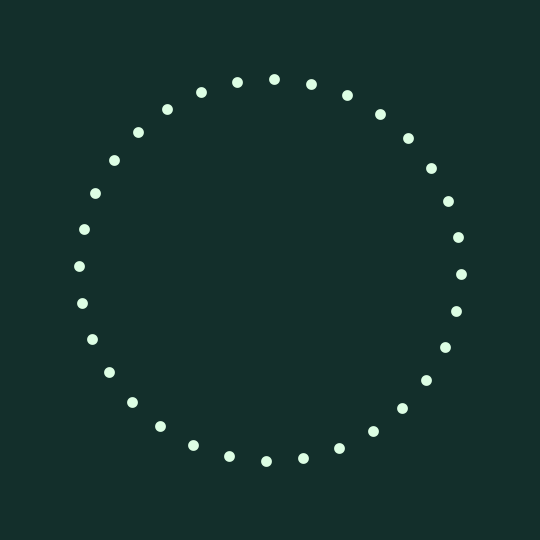
I start with 32 equally spaced points on the equator of the sphere. Now, rotate these points around the axis $(1,0,1)$ and project back down to the equator along the meridian passing through the point: the result is the above animation (after dropping the last coordinate and treating the resulting points as living in the plane).

And here's the full 3-D picture showing the rotations and the projection map; the dark points are the rotating points, the light points are the points on the equator that I'm projecting to, and the thin lines show the meridians:

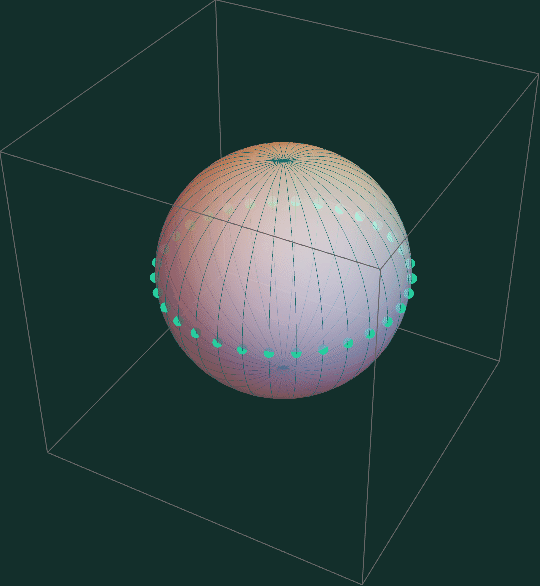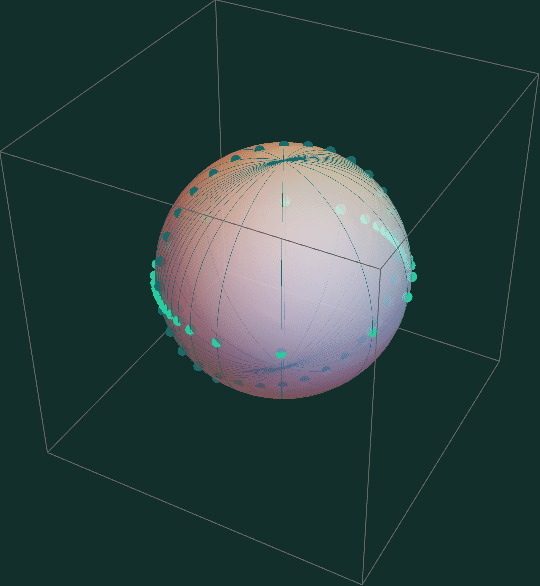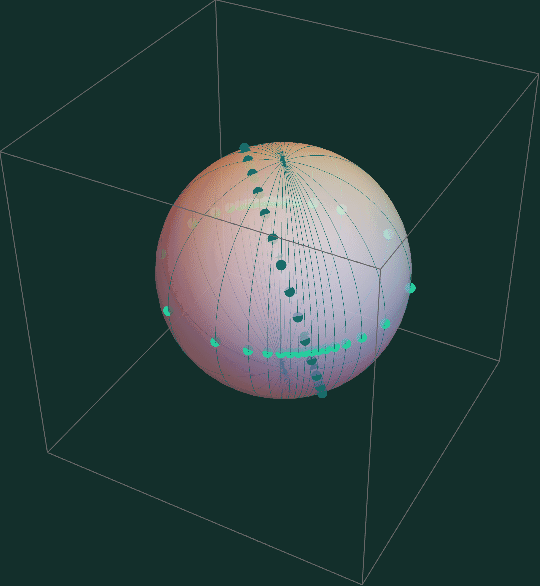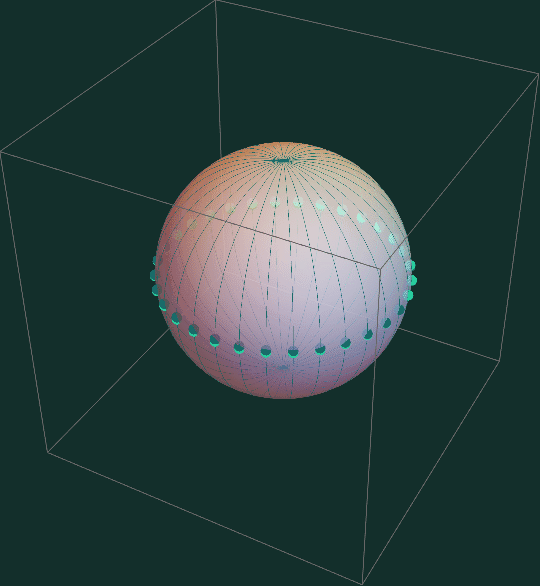
Source code:

SphereToCircle[p_] := {Cos[#], Sin[#], 0} &[ToSphericalCoordinates[p][[3]]];

RotateAndProject[p_, ?_, w_] := SphereToCircle[RotationTransform[?, w][p]];

With[{n = 32, cols = RGBColor /@ {"#DDFEE4", "#132F2B"}},
 Manipulate[
  Graphics[
   {PointSize[.02], cols[[1]],
    Point /@ 
     Table[RotateAndProject[{Cos[?], Sin[?], 0}, t, {1, 0, 1}][[;; 2]], 
        {?, 0, 2 ? - 2 ?/n, 2 ?/n}]},
   PlotRange -> Sqrt[2], ImageSize -> 540, Background -> cols[[-1]]],
  {t, 0, 2 ?}]
 ]