Try this code. There are two typical integrals through which one can express a solution
In[1]:= r0 = {x0, y0, z0};
r[1] = {x, W/2 - t*W, T/2};
r[2] = {x, -W/2, T/2 - t*T};
r[3] = {x, -W/2 + t*W, -T/2};
r[4] = {x, W/2, -T/2 + t*T};
rt = Table[D[r[i], t], {i, 1, 4}]
Out[6]= {{0, -W, 0}, {0, 0, -T}, {0, W, 0}, {0, 0, T}}
In[7]:= B =
Sum[Cross[rt[[i]], (r[i] - r0)]/((r[i] - r0).(r[i] - r0))^(3/2), {i,
1, 4}]
Out[7]= {(-((T W)/2) -
T y0)/((x - x0)^2 + (-(W/2) - y0)^2 + (T/2 - t T - z0)^2)^(
3/2) + (-((T W)/2) +
T y0)/((x - x0)^2 + (W/2 - y0)^2 + (-(T/2) + t T - z0)^2)^(
3/2) + (-((T W)/2) -
W z0)/((x - x0)^2 + (-(W/2) + t W - y0)^2 + (-(T/2) - z0)^2)^(
3/2) + (-((T W)/2) +
W z0)/((x - x0)^2 + (W/2 - t W - y0)^2 + (T/2 - z0)^2)^(
3/2), (-T x +
T x0)/((x - x0)^2 + (-(W/2) - y0)^2 + (T/2 - t T - z0)^2)^(3/2) + (
T x - T x0)/((x - x0)^2 + (W/2 - y0)^2 + (-(T/2) + t T - z0)^2)^(
3/2), (-W x +
W x0)/((x - x0)^2 + (-(W/2) + t W - y0)^2 + (-(T/2) - z0)^2)^(
3/2) + (W x -
W x0)/((x - x0)^2 + (W/2 - t W - y0)^2 + (T/2 - z0)^2)^(3/2)}
In[23]:= Integrate[Integrate[1/(p^2 + u^2 + v^2)^(3/2), u], v]
Out[23]= ArcTan[(u v)/(p Sqrt[p^2 + u^2 + v^2])]/p
In[9]:= Integrate[Integrate[v/(p^2 + u^2 + v^2)^(3/2), u], v]
Out[9]= -ArcTanh[Sqrt[p^2 + u^2 + v^2]/u]
In[10]:= I1[p_, u_, v_] := ArcTan[(u v)/(p Sqrt[p^2 + u^2 + v^2])]/p
I2[p_, u_, v_] := -ArcTanh[Sqrt[p^2 + u^2 + v^2]/u]
In[17]:= Bx4[x_, t_, x0_, y0_, z0_, W_,
T_] := -(W/2 + y0)*
I1[W/2 + y0, t*T + z0 - T/2, x - x0] + (y0 - W/2)*
I1[y0 - W/2, t*T - z0 - T/2, x - x0] - (z0 + T/2)*
I1[z0 + T/2, t*W - y0 - W/2, x - x0] + (z0 - T/2)*
I1[z0 - T/2, t*W + y0 - W/2, x - x0]
In[18]:= Bx2[x_, x0_, y0_, z0_, W_, T_] :=
Bx4[x, 1, x0, y0, z0, W, T] - Bx4[x, 0, x0, y0, z0, W, T]
In[19]:= Bx[x0_, y0_, z0_, W_, T_,
L_] := (Bx2[L/2, x0, y0, z0, W, T] -
Bx2[-L/2, x0, y0, z0, W, T])/(4*Pi)
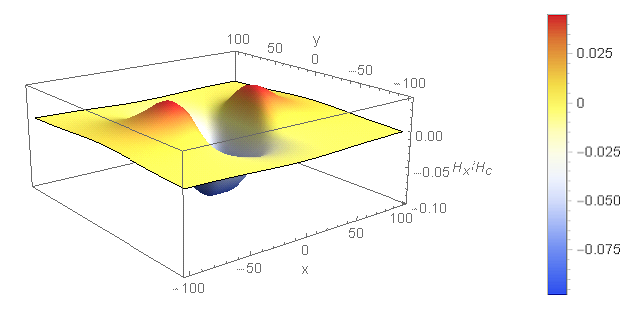
In[35]:= Plot3D[-Bx[x, y, 20, 50, 20, 50], {x, -100, 100}, {y, -100,
100}, Mesh -> None, ColorFunction -> "TemperatureMap",
PlotLegends -> Automatic,
AxesLabel -> {"x", "y",
"\!\(\*SubscriptBox[\(H\), \(x\)]\)/\!\(\*SubscriptBox[\(H\), \
\(c\)]\)"}, PlotRange -> All, PlotPoints -> 50, Exclusions -> None]
 Attachments:
Attachments: