Hello to everybody,

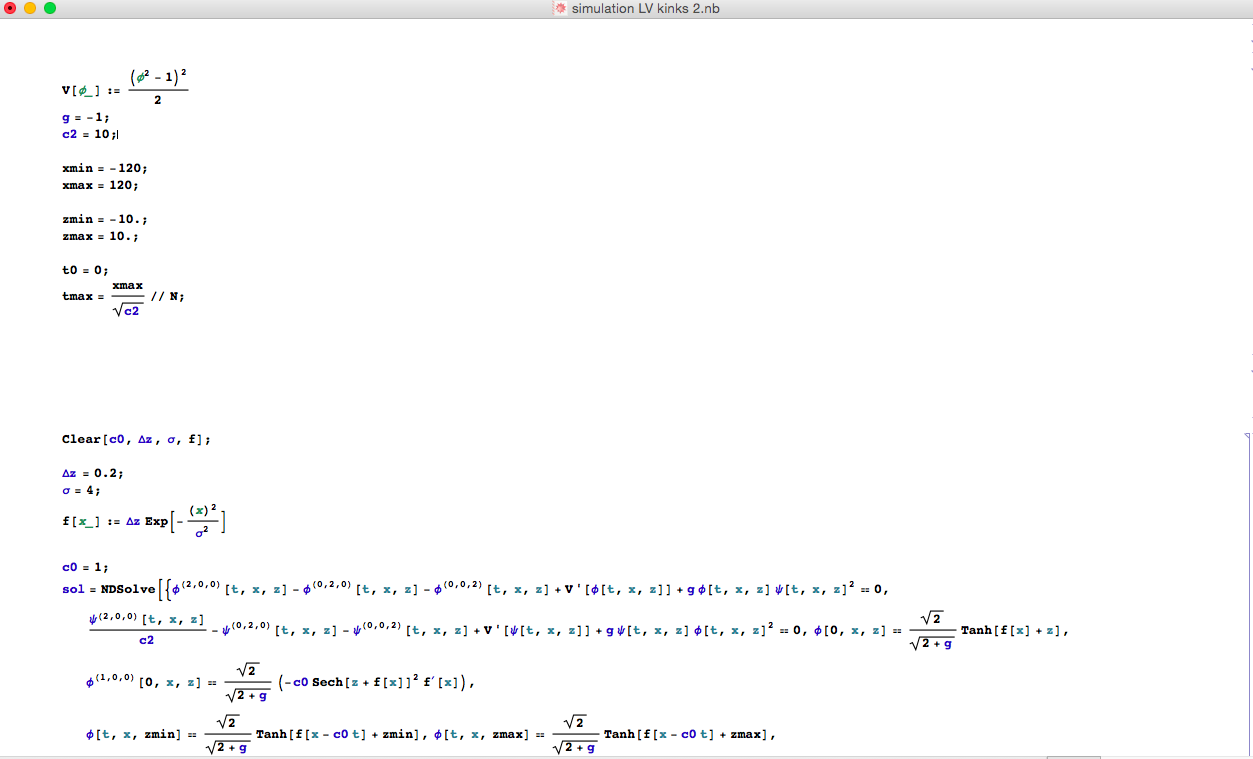
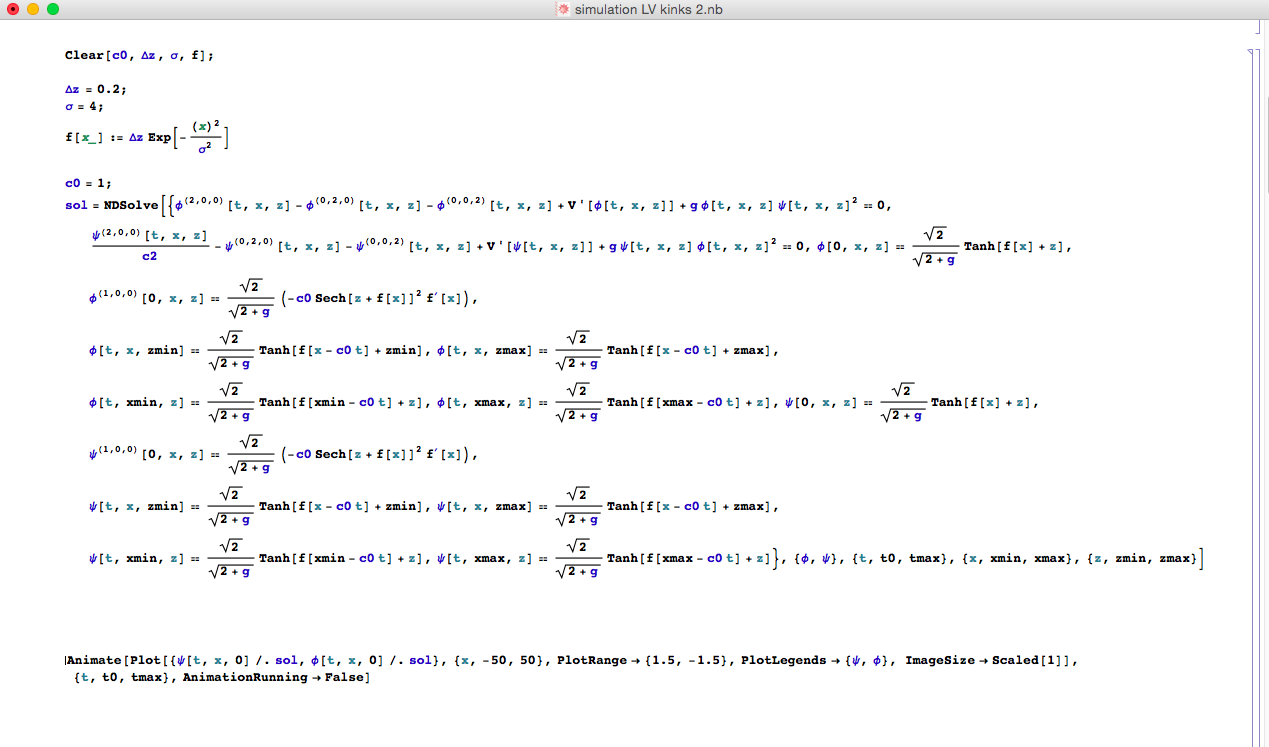
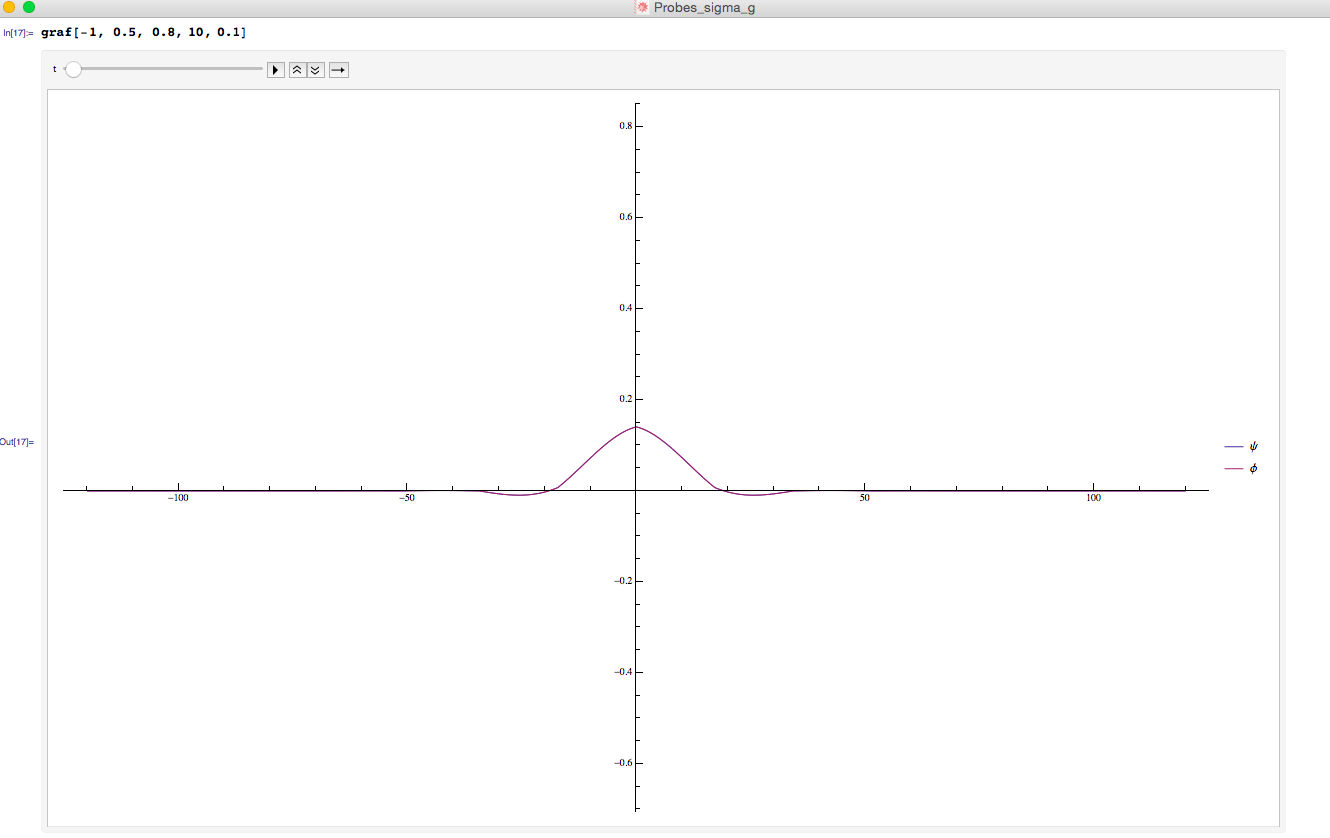
I was trying to solve a couple of differential equations with NDSolve, they are functions of "x", "z" and the time "t". As a initial condition I impose the function to be the Hyperbolic tangent of a gaussian at t = 0, but to my surprise when I use the function "Animate", at t = 0 the function is not exactly a the hyperbolic tangent of a gaussian but rather something close to it. Why this is so? Is it possible that Mathematica changes "arbitrarily" the inital conditions in order to make it easier to solve???

Any suggestion??

I attached the screenshots of the code I use to arrive to the solutions and the plot of the supposed Tanh of the Gaussian too. You will see is not exactly the Hyperbolic tangent of a gaussian.

Thanks a lot in advance!