Here are some musings on the subject. There are many famous objects available in Wolfram Language as built-in data. For example:
RegionProduct[
DiscretizeGraphics[
Entity["Lamina", "BatmanInisgniaLamina"][EntityProperty["Lamina", "BoundaryDiagram"]]
],
BoundaryMeshRegion[{{0},{3}},Point[{{1},{2}}]]
]
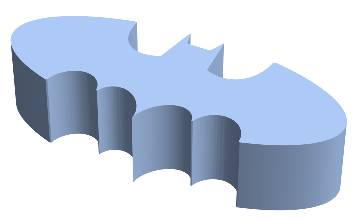
You can get many properties:
EntityProperties["Lamina"]
If you would like to deal with custom curves, not present in the built-in data, first assign your plot to a variable:
batPLT=Plot[{
With[{
w=3*Sqrt[1-(x/7)^2],
l=(6/7)*Sqrt[10]+(3+x)/2-(3/7)*Sqrt[10]*Sqrt[4-(x+1)^2],
h=(1/2)*(3*(Abs[x-1/2]+Abs[x+1/2]+6)-11*(Abs[x-3/4]+Abs[x+3/4])),
r=(6/7)*Sqrt[10]+(3-x)/2-(3/7)*Sqrt[10]*Sqrt[4-(x-1)^2]},
w+(l-w)*UnitStep[x+3]+(h-l)*UnitStep[x+1]+(r-h)*UnitStep[x-1]+(w-r)*UnitStep[x-3]],
(1/2)*(3*Sqrt[1-(x/7)^2]+Sqrt[1-(Abs[Abs[x]-2]-1)^2]+Abs[x/2]-((3*Sqrt[33]-7)/112)*x^2-3)*
((x+4)/Abs[x+4]-(x-4)/Abs[x-4])-3*Sqrt[1-(x/7)^2]},
{x,-7,7},AspectRatio->Automatic];
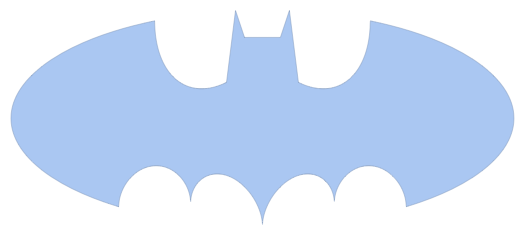
Get only the points of the plot and make sure they are sorted properly. Then build a flat mesh region:
points = Flatten[MapAt[Reverse, Cases[batPLT, Line[x_] -> x, Infinity], 1], 1];
batREG = BoundaryMeshRegion[points, Line[Append[Range[Length[points]], 1]]]
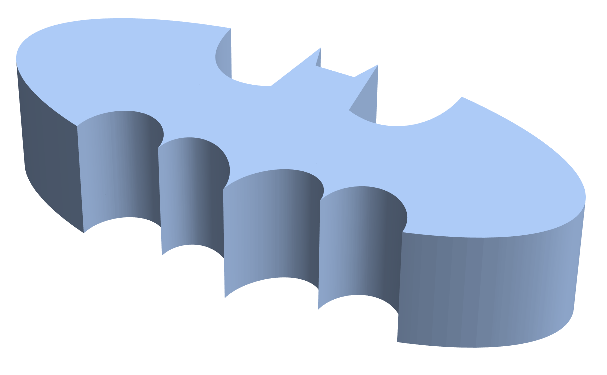
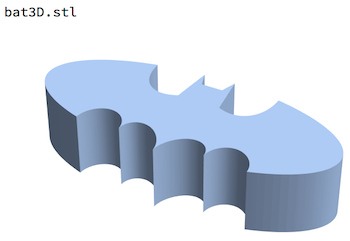
You can extrude it to 3D as:
bat3D = RegionProduct[batREG, BoundaryMeshRegion[{{0}, {3}}, Point[{{1}, {2}}]]]
Next export to a 3D printing format and verify that export indeed worked:
Export["bat3D.stl", bat3D]
Import[%]
There could be a better way to do this, perhaps suggestions will follow. But don't wait, read documentation about all functions used above and explore on your own.