Group Abstract Group Abstract

Message Boards Message Boards

0
|
7.2K Views
|
2 Replies
|
1 Total Like
View groups...
Share
Share this post:

Solve the following integrals with assumptions?

Ox Clouding
Posted 5 years ago

Hi everyone,

I have come across this difficult integral today:

int1=Integrate[Sqrt[1 - s^2]/(x - s), {s, -1, 1}, 
 Assumptions -> {-1 < x < 1}]

It's the x derivative of the following function int2, which numerical integration indicates to be finite and continuous (but the numerical integration have some issues too, as shown): 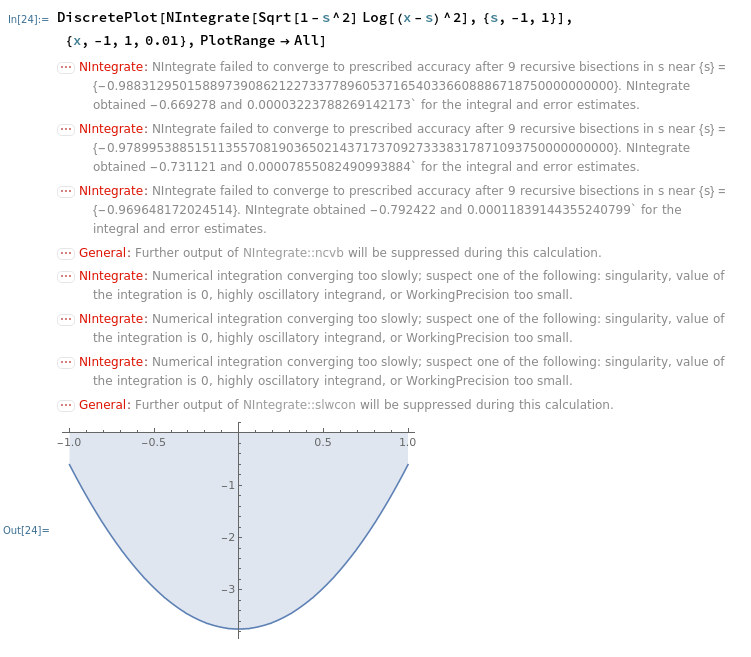int1=dint2/dx

I have also tried to do numerical integration of the original integral, but the result is weird: enter image description here

I know adding "GenerateConditions->False" will help MMA to give an output:

Integrate[Sqrt[1 - s^2]/(x - s), {s, -1, 1}, 
 Assumptions -> {-1 < x < 1}, GenerateConditions -> False]

Gives output Pi x. But since I arbitrarily removed the conditions checking in MMA so I am not sure if this is the proper way doing it.

Is there any better way to evaluate either int1 or int2 in Mathematica?

Thanks in advance!
POSTED BY: Ox Clouding
2 Replies
Sort By:
Ox Clouding
Ox Clouding
Posted 5 years ago

Dear Daniel,

Thank you for the reply. I have found the answer myself:

Integrate[Sqrt[1 - s^2]/(x - s), {s, -1, 1}, 
 Assumptions -> {-1 < x < 1}, PrincipalValue -> True]

will give the output Pi x. I think it's related to something about being an improper integral but I am not knowledgeable enough to give a full explanation. By the way, int2 can also be done in the same way but the result is much complicated: enter image description here And it rises non-zero imaginary parts which should not be there due to the physical nature of this integral, but again I can't give a better explanation.

I hope there are some ways to evaluate int2 directly and generate a real parabolic solution (similar to the results from numerical integration, which is also the analytical result from the reference). But being able to evaluate int1 is good enough already. So thanks anyway!
POSTED BY: Ox Clouding

Here are smaller examples eliciting the behavior.

(* In[48]:=*) Integrate[Log[x - s], {s, 0, 1}, Assumptions -> 0 < x < 1]

(* Out[48]= -1 - I \[Pi] (-1 + x) - (-1 + x) Log[1 - x] + x Log[x] *)

(* In[49]:= *)Integrate[D[Log[x - s], x], {s, 0, 1}, 
 Assumptions -> 0 < x < 1]

(* During evaluation of In[49]:= Integrate::idiv: Integral of 1/(-s+x) does not converge on {0,1}.

Out[49]= Integrate[1/(-s + x), {s, 0, 1}, Assumptions -> 0 < x < 1] *)

Both are behaving correctly What they show, in essence, is that a function's antiderivative can behave better than the function..
POSTED BY: Daniel Lichtblau
Reply to this discussion
Community posts can be styled and formatted using the Markdown syntax.
Tag limit exceeded
Note: Only the first five people you tag will receive an email notification; the other tagged names will appear as links to their profiles.
Reply Preview
Attachments
Remove
or Discard