I don't know the reason. What your latest examples show, however, is more "global" variation across the entire grid. The Null isn't causing unusual padding. The presence of the Null is causing the GraphicsGrid to calculate it's dimensions differently, but once it's done that, the result is "regular".

As Rohit said, it seems to be harder to control the output with GraphicsGrid than with Grid. I haven't used GraphicsGrid much, so I don't have any suggestions for how to get what you want. You'll have to play around with the options (e.g. Alignment). Or just use Grid.

However, if you're wanting each "cell" in the graphics grid to have the same dimensions, then you're never going to regularize the padding if your plots have different dimensions. Space will have to be added somewhere. So, maybe you can play with the Plot options for each little plot so that they are all the same dimensions and no variations in padding will occur.

Pick your poison. (That's an English idiom that means none of the choices are ideal. It's a dilemma. You'll have to choose the least bad option.)

Here, this should illustrate what I'm saying:

plots = 
  Table[
    Plot[
      k Sin[x], {x, 0, 2 Pi}, AspectRatio -> Automatic, Background -> LightBlue], 
      {k, 1, 6}];
GraphicsGrid[Partition[plots, 3], Dividers -> All]

Okay. Your hypothesis is that in a GraphicsGrid, the presence of a Null in one "cell" of the grid causes extra spacing to occur in an adjacent "cell". Let's test that:

b = Table[Plot[Sin[x], {x, 0, 3}], {b, 5}];

Now, let's try all six possible positions for Null:

GraphicsGrid[Partition[Insert[b, Null, 1], 3], ImageSize -> Full]

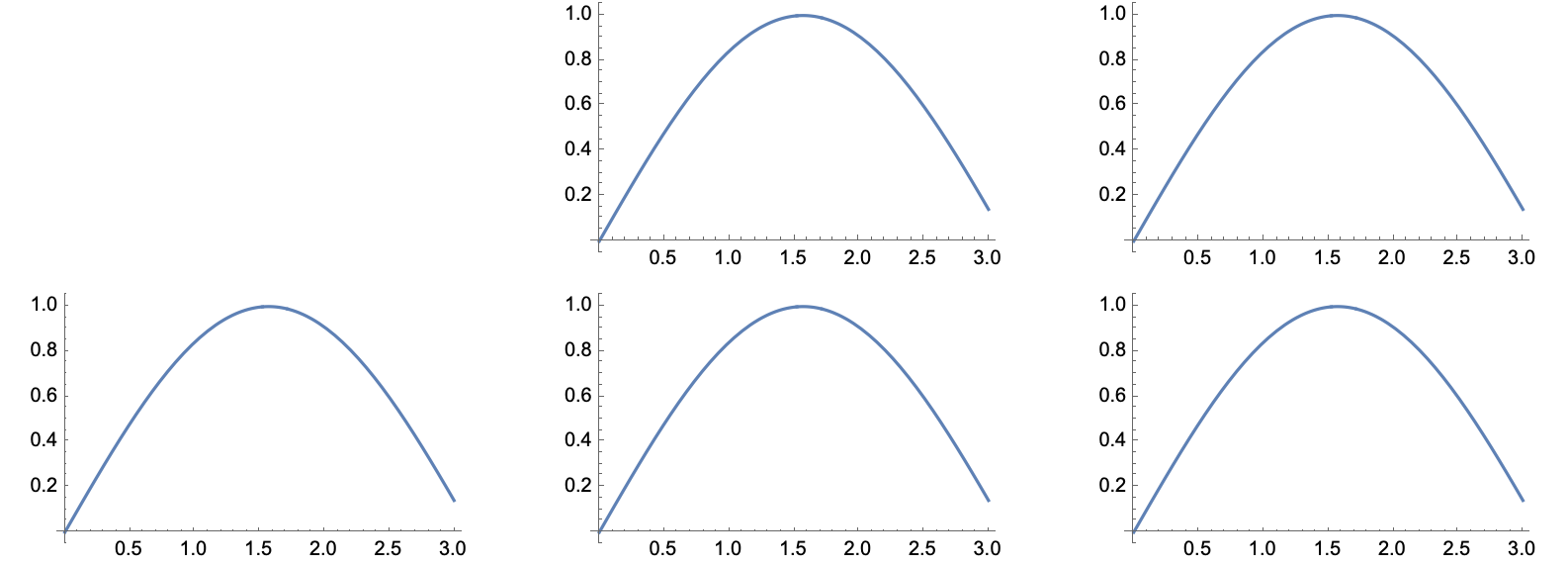
GraphicsGrid[Partition[Insert[b, Null, 2], 3], ImageSize -> Full]

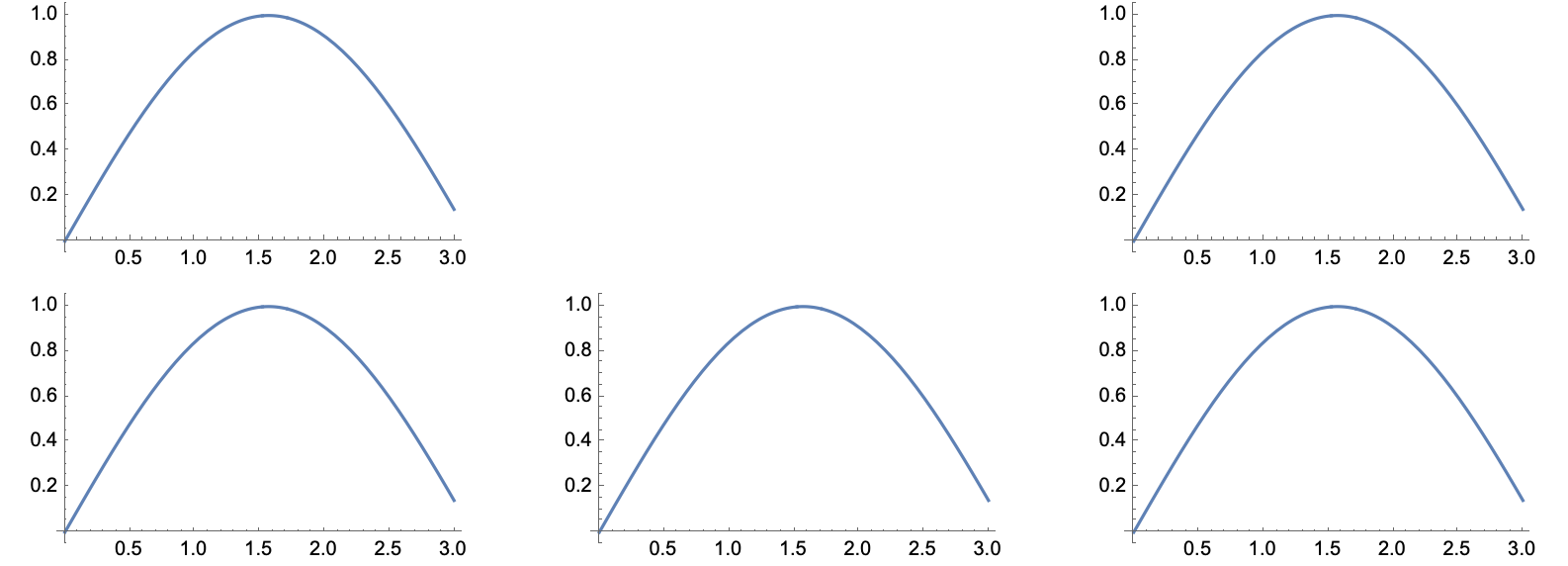
GraphicsGrid[Partition[Insert[b, Null, 3], 3], ImageSize -> Full]

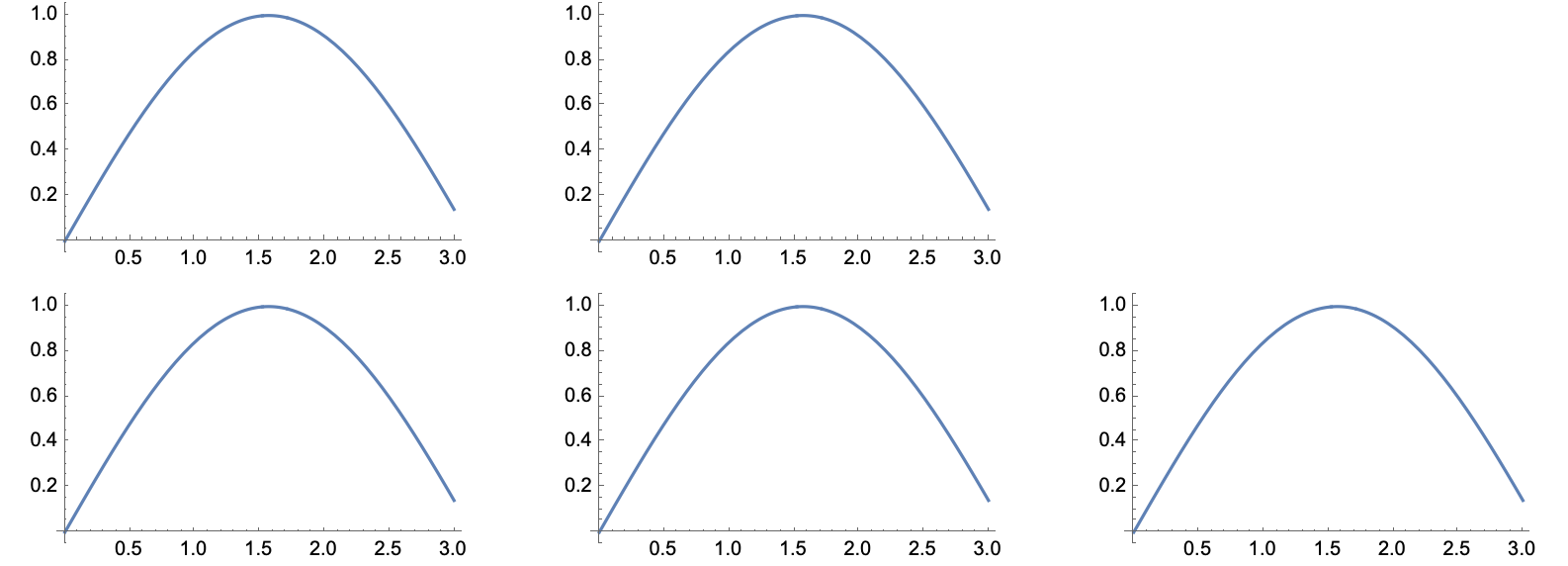
GraphicsGrid[Partition[Insert[b, Null, 4], 3], ImageSize -> Full]

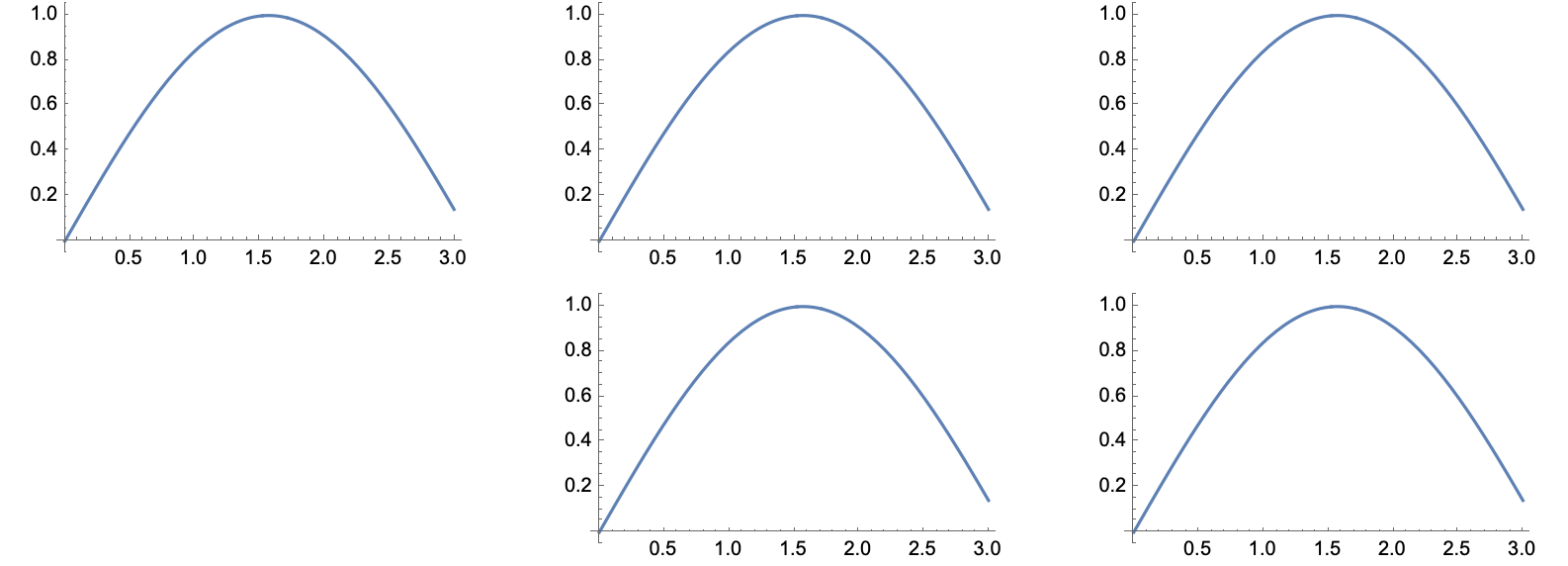
GraphicsGrid[Partition[Insert[b, Null, 5], 3], ImageSize -> Full]

GraphicsGrid[Partition[Insert[b, Null, 6], 3], ImageSize -> Full]

Your eyes are probably better than mine. Please point out to me which one of these images shows that extra space was added. If the spacing in these images was not affected by Null, then what could have affected the spacing in your original example? It seems like a reasonable conclusion to me that it has something to do with scaling different images to fit the grid.

Furthermore, your examples actually support MY hypothesis. In your latest example, you show one grid where padding seemed to be added and one where it was not. In the grid with extra padding, it was placed in a row where one image had tick marks at the bottom and the rest did not. In the grid with no extra padding, every element in each row of the grid was the same in this regard. Furthermore, in the second grid, PADDING WAS ADDED EXACTLY AS I PREDICTED. The padding between ROWS is now larger. You don't seem to care about that spacing, but it is there. The horizontal padding is due to one of the plots having a thinner aspect ratio. The vertical padding is due to 5 of them having wider aspect ratio.

Null changes the space around the figure:

a = Table[Plot[Sin[x], {x, 0, b}], {b, 5}];

b = GraphicsGrid[Partition[Prepend[a, Null], 3], ImageSize -> Full]

c=GraphicsGrid[Partition[a, UpTo[3]], ImageSize -> Full]

Because when forming a graph, I want the upper left corner to be empty instead of the lower right corner. Wherever to be empty with Partition, it does not has the same gaps between figures as no Null.

But Grid can not align axes and When the images are large, the grid will make the images too small.