Hi.

Being not completely sure if this is the right forum for this kind of questioning, I approach you with gratitude of time and attention.

I don't know math, nor physics, neither do I know anyone who does. I'm a young architect and have been lately perplexed and bound to the theories of an elemental structure for the universe, as well as to the happenings that took place before / at the big bang.

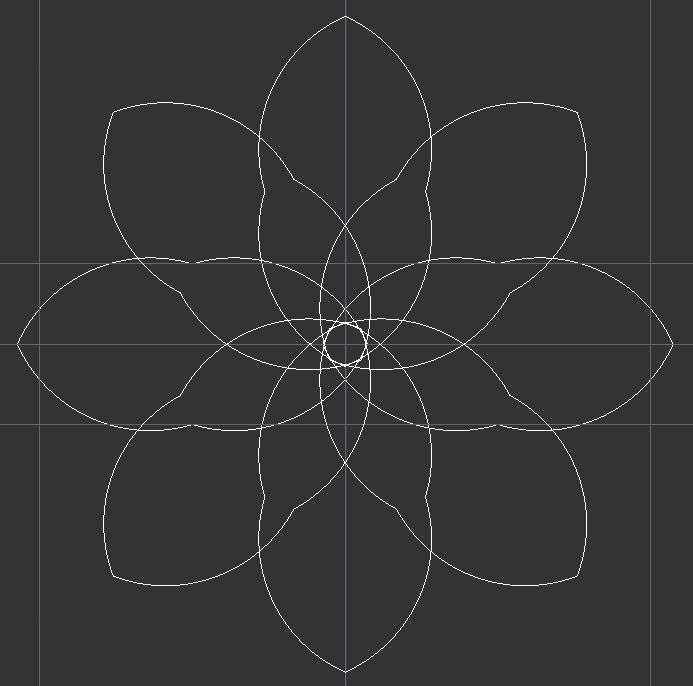
So trough merely fantasia and frustration to visualize / comprehend the hyperspace; i traced some curves to the best i could retrieve is a Brachistochrone curve, this to make sketches of a structure, out of these modules; made from the mirroring of two conjoining said curves 1 (4 total per module). I decided on the criteria for the array and the origin (¿origins?) by a line of thought for a time minimizing trajectory ¿in all directions?

Below; four curves [1], form a module

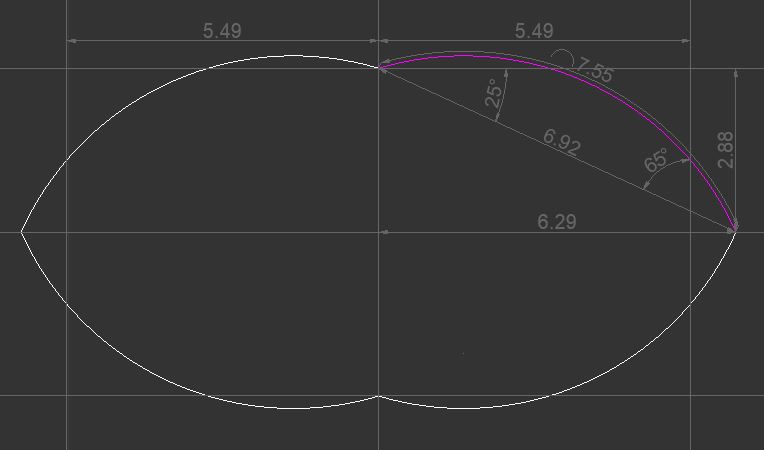
Below, two modules mirrored

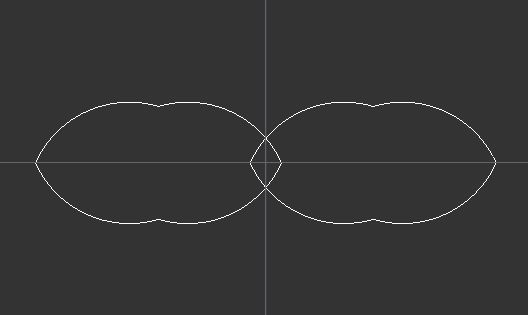
From the center; we continue on adding modules in a ¿symmetric matrix manner?. I have no clue as to how the starting condition could evolve to the structure proposed... This would be the basis of my questioning...

Below; group of two modules mirrored 90° from the center of the graph, creates a group of four modules

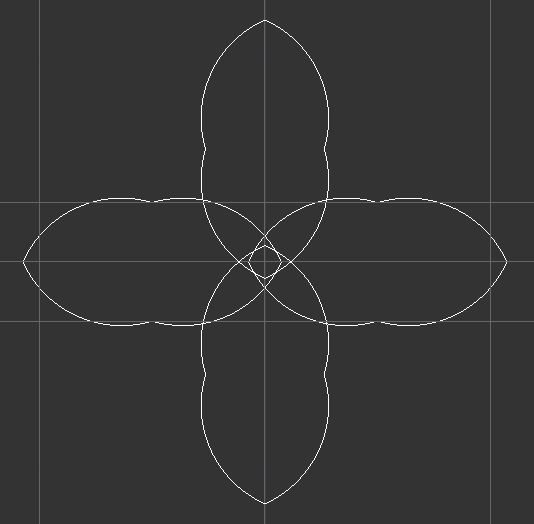
…How can the paths and connectors yield continuity for the structure as we continue on adding modules? Maybe not even as modules, but as individual path connectors that througoth their interactions gradually give form to the module, later to a cell; in a constant, continuous and constructive manner. Also; ¿cyclical?

¿Can one even graph in curves? I really don't know.

Now, a group of four modules like the one above is rotated 45° to be juxtaposed on the graph...

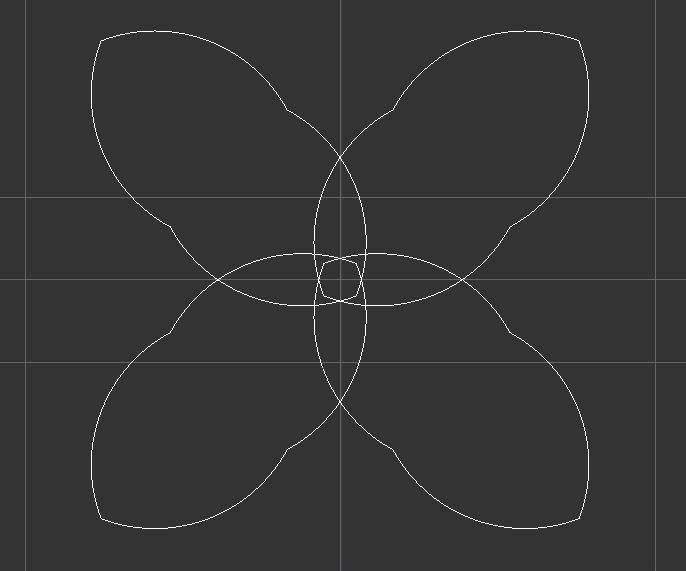
After the juxtapositioning mentioned before, below on "Image 8"; we end up with what we'll call a basic cell. A total of 8 modules in a polar array from the center of the graph.

Using the word juxtaposition; it refers to the connections between the modules in different orientations, not considering them would not mean that they're not there. For it would be required that their vertices and/or interactions are connected on the complex plane.

¿Could this be through the tracing of a "guide" structure?

"Image 8"

This attempt of establishing a rule; will be the criteria for the structure proposed. From now on; the graph will yield bigger and bigger basic cells in the same number of steps. (¿rule?)

The basic cell produced by the modules would now itself become a module; on its way to form a basic cell.

Below. the previous basic cell mirrored by the center to a group of two modules.

Highlighted; particularities observed.

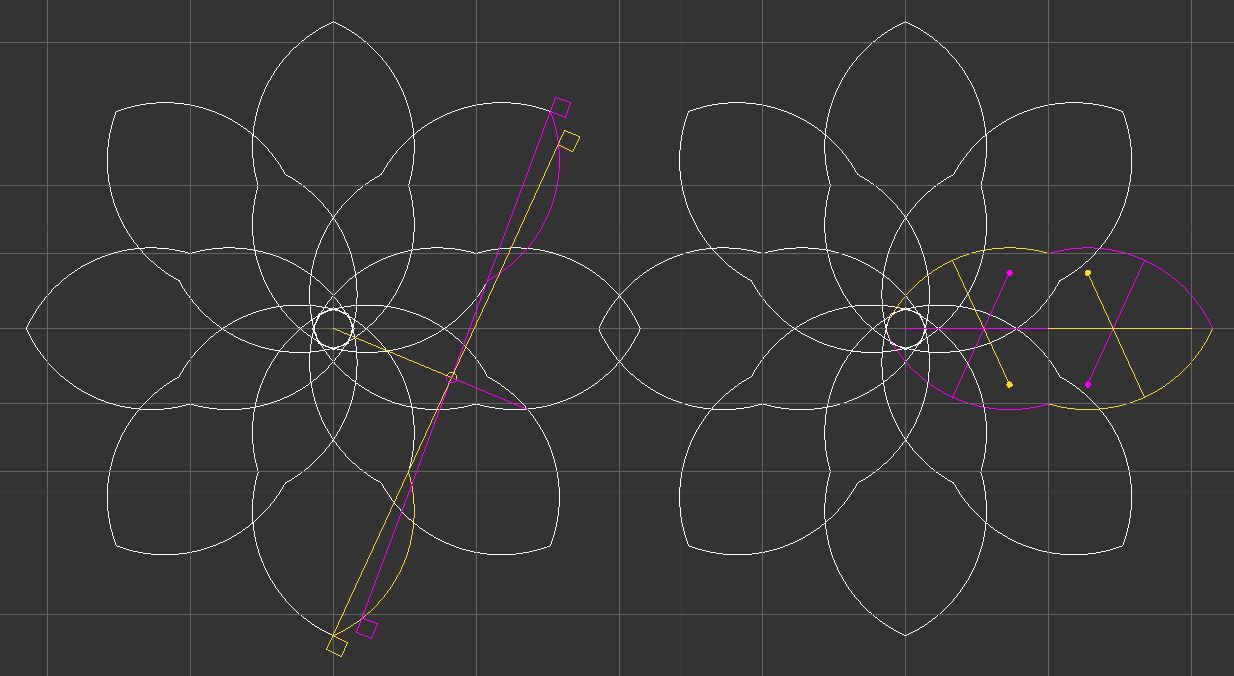
Particularities on left module: 
In pink and yellow; planes that respectively yield a Brachistochrone curve.

¿Is it something; that the planes onto which the cycloids traverse to yield a Brachistochrone, intersect directly with the axis of intersections for neighbor curves?

Particularities on right module: 
In pink and yellow; centers for the rods and respective trajectories
(curves and their radius)

¿Is it something; that the intersections from the rods on equilibrium for their respective trajectories, intersect with the central axis for the graph?

This questions are not meant to point something out neither do they have to be answered; they come up as the structure is proposed. It is so, that; to this point I've tried to make it obvious but will now make it explicit.

I have no idea what I'm talking about.

We'll continue to see the structure as i explain. Below, the following steps to the ¿rule?.

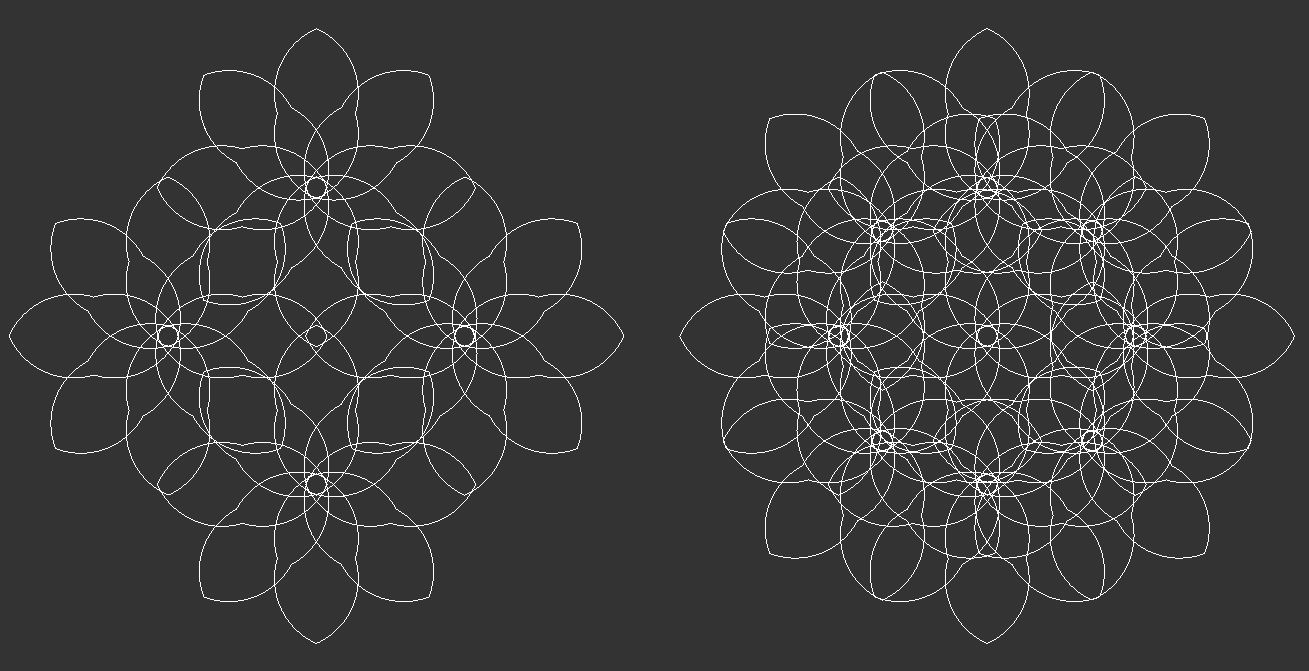
    On the left: 

The previous group of two modules is mirrored 90° to a group of four modules.

    On the right: 

Now our group of four modules rotates 45° to be juxtaposed, creating our "basic cell" out of eight modules.

And so on...

In the next image (img.12) we'll see what would be our previous "basic cell"; turned through the ¿rule? into modules (eight), and onto our basic cell, again.

Now explaining on; the approach to the structure is no more, than an exercise, it started with doodles on the back of documents and it went on into AutoCAD, which the images of the structure shown; are from...

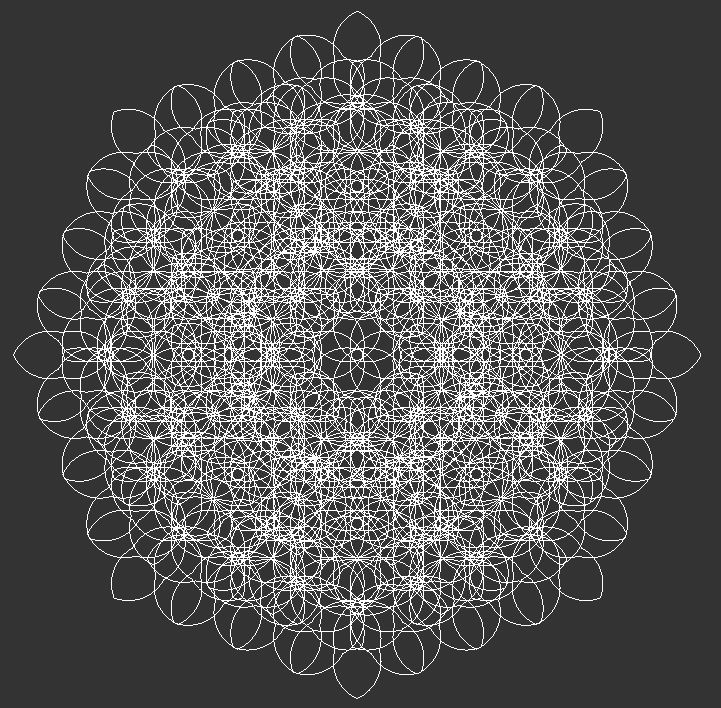"Image 12"

And so, as i continued to torture the integrated graphics card on my laptop; by repeating the ¿rule? to the structure. I began to notice asymmetries and irregularities, also; it started to get harder and harder to operate through high quantities of geometry. The generating of images for observing as the scaling of the structure became an issue as well.

It was then that i ended up in Wolfram cloud; to try and make a mathematical representation.

I failed tremendously...

Above we see attempts to graph or plot; ¿something?.

At least i learned about monads…

That brought us to this moment (hi again) on this community.

And as this turns more into a narrative than an idea or question; i do come to you for help.

In the Wolfram cloud i encounter a world of information, i got lost in the info; no idea of how to start or how to even classify it. I do not even know if it is a Hamiltonian graph as i said in the title.

Does it has to do with; Euler equations? .. Jacobi amplitude? .. Is it a cycle graph? is it a circulant graph? .. is it a wheel graph? .. star graph? .. complete graph? .. can it even have connectivity? .. is it finite? .. infinite? .. is it a matrix? .. Fourier series? .. Cohomology? .. Hodge theory? ..
i'll stop now.

What I'm getting to is that I'm not expecting that this gets graphed for me, nor that my random questions get answered.

Before we see the visual results of the exercise, we'll do two things.

        Thing 1 - Pose concrete questions. As well as a challenge. 

Graph the structure proposed.

1.- ¿What could be a rule for the graph?

1.1 - As for an equal number of steps that yield constant exponential growth.

2.- ¿Feasible starting condition?

2.2 - Considering the brachistochrone curve as the fundamental structure for our modules; direction of edges, paths to evolution and connectivity overall as a hyperstructure.

3.- ¿On which field(s) would this venture fall in to? 

3.3 - Theorems, functions, equations, matrices or themes to look into for concrete information on the established objective.

Bonus:

B-1 - Origin / Center:

B-1.1 - Up until now I've been referring to an "origin(s)" or "center of the graph" only to mark a reference. For the exercise, as the steps went on; the center kept on moving depending on where one would decide to repeat the ¿rule? (the polar array).

Ideally the structure would have to evolve in a multi-directional proportional manner.

B-1.2 - ¿Would the graph have a center / origin or starting point; could the evolution only start with a path from A to B posing no specific start point but connections?

B-2 - Complex Plane

B-2.1 - Previously I've mentioned the complex plane. I have yet to understand the application and definition for this.

B-2.2 - ¿How many dimensions would our structure correspond to or demand; if permitted by the criteria; we added planes and dimensions (say Z): which would be the min-max number of coordinates needed to specify a position?

-¿Can the structure posed even be represented as a hyperstructure?

B-3 -¿Could this structure be in any way a representation of Physics?

Given the nature of the Brachistochrone; could we end up representing a phenomena or a relation to any basic property of Physics. Eg. Light refraction, electromagnetic fields (I'm dreaming here).

B-4 - ¿Is it even something to trace Brachistochrone curves as an underlying basis? 
B-5 - ¿Something like this has already been graphed?

           Thing 2 - Philosophical argument.

An interpretation.

I'll attempt to pose the fundamental ideas on which the structure was conceived.

With an emphasis in the fun nature for the exercise.

A.K.A: Do not take this as an educated nor grounded opinión / proposal. This is a fever dream.

A) Rescued from The Brachistochrone, with Steven Strogatz a video from the YouTube channel 3Blue1Brown, posted by Grant Sanderson 04/01/16.

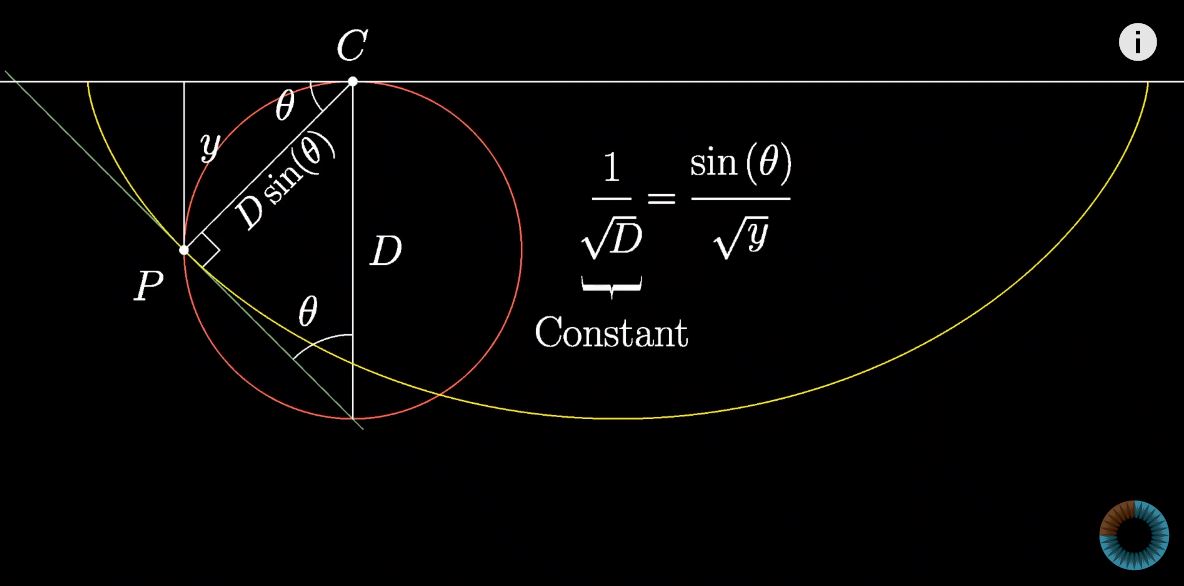
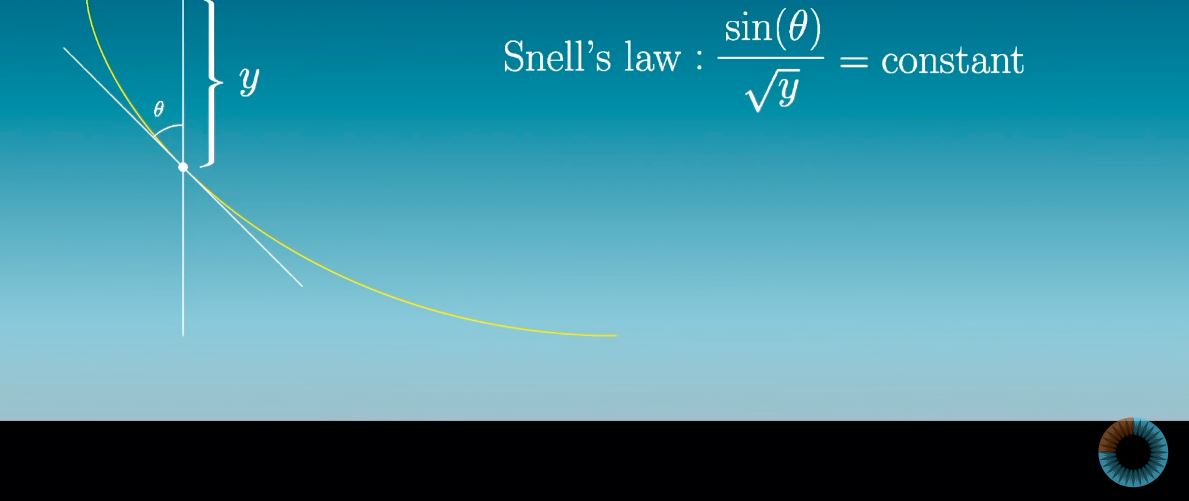
Grant tells us how drawing a line perpendicular to the plane of a cycloid to a tangent point on the curve (y) is equal to 1/√D=sin(θ)/√y .. Which for the diameter of the circle that stays constant throughout the cycloid; the relation will be constant; later a connection to Snell's law.

Then moves on to examine the equations for a cycloid and to extend a challenge...

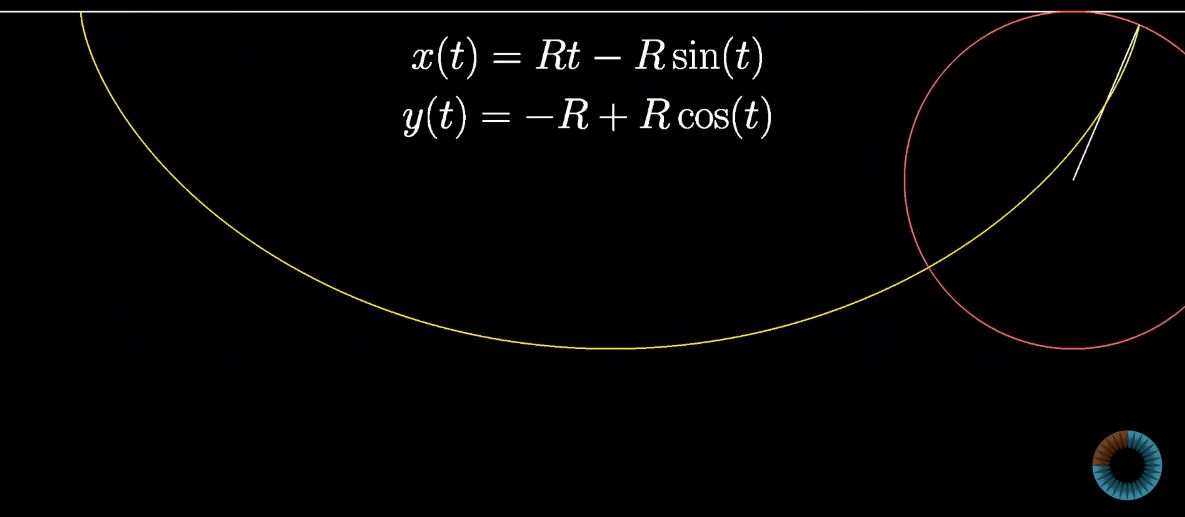
For the equations:

Questions the position of an object along the curve as to a function of time.

-¿Would time start slowly in incremental speed?

-¿If so, as to what function?

"-It turns out the cycloid will turn at a constant rate. Gravity pulls the object along the curve precisely as a rotating wheel (cycloid) would"

This means the position; as a function of time, for our hypothetical object on the curve, would be a straight line; described not by [x(t), y(t)], but as [θ(t)] .. for the angle that the velocity vector makes (θ) as for a function of time.

Below, Solutions to the Brachistochrone problem seen as for the [x(t), y(t)] plane.

Below, Solutions to the Brachistochrone problem seen as for the [θ(t)] plane. -"θ increases at a constant rate with respect to t".

The animation in 3Blue1Brown's video it's much more dynamic.

For 3Blue1Brown Brachistochrone challenge:

Further ahead we'll come back to this challenge..

An intuitive reason.

   Philosophical argument.

B) Imagine; our structure beginning from what we'll call an event, this takes place on infinite empty space.

Structure: Any totality of events.

Event: Picture a conic section at the vertex, parallel to the directrix; a point. Then, this; acting as a motion forward on all directions in the most efficient use of space.

Introduce Duality; *to be, there has to be; the not to be.*

Depiction of our current definition of event is shown below.

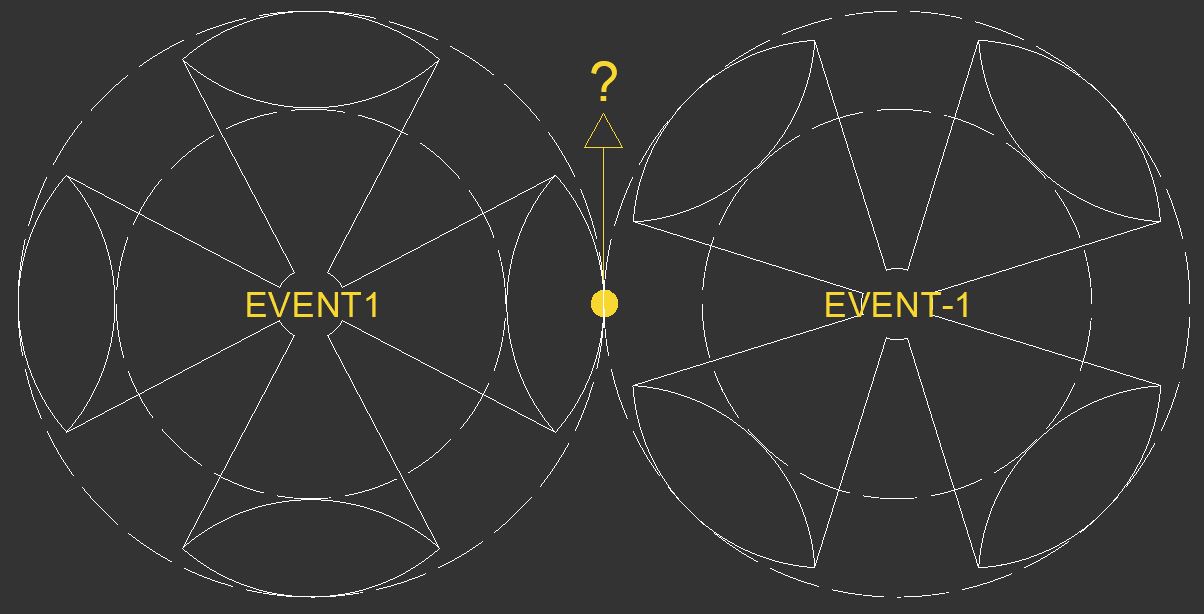The geometry in this image is a graphic depiction of a concept, bears no other relation to the structure.

Consider: Supersymmetry, Multiverse; let us assume then the following.

At the existence of an event, there for to be a structure; an opposite event has to take place..

In the existence of multiple events we would have then; respectively multiple opposite events.

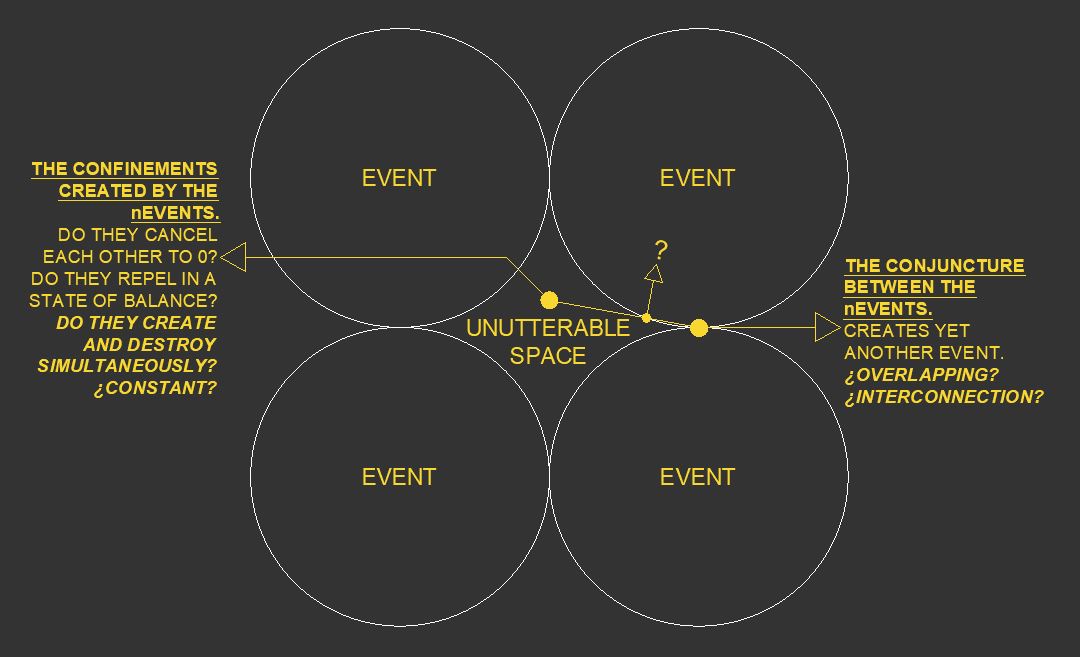The geometry in this image is a graphic depiction of a concept, bears no other relation to the structure.

Accounting for Singularity in relation to inflation; we imagine:

-The "Event" takes place, there for; n events take place simultaneously. The events interact with each other; themselves events. This yields a total geodesic event.

These motions could then; proceed forward to a point of encounter (picture two blasts of water colliding at the same axis). The encounter of multiple events would then inevitably build limits, carried out to diverse circumstances dependent on their particularities, this on a cycle of competition for space.

Imagine this getting to a confinement, as inwards like outwards to underlying.

"Image 38" .. Above; development of the structure to an unregistered number of steps. As mentioned before; i cannot account for regularity to a rule.

Abstractions of the exercise below...

"Image 39" .. We can refer to Image [4] on which these concepts are here abstracted.

...For the most efficient path… All of the events would arrive to an interaction at the same instant, sharing space in a symmetrical arrangement...

Most left centered close-up of img.[38]

We can describe the image above as en edge of the structure; as for the state of development shown on "Image [38]". The sum of edges enclose a basic cell. From this perimeter the ¿rule? would repeat.

...At this interactions one could infer a number of situations, for a given; a new event that for its nature of being a result of previous happenings; takes place at a different instant...

"Image 45" .. Above; development of the structure a step further than Image [38], the frame and position are the same. This leads the image to saturation, still; we can appreciate small details that show for remaining space, this also shows in which direction the steps were carried on; which would be the more saturated areas (left side).

...At this result; of a new event, it could be that the motions are constantly in eternal friction or fusion; now interlocked to the new event. It could be as well that the new event acts as a counter motion, but then again; these counter motions would as well come to a point of encounter...

"Image 46" .. "Most left centered close-up of img.[45]"

...Be that as it may; this situation would describe some sort of symmetrical cycle or constant state for creation and destruction of space and structure.

For a case scenario in which our structure reached a boundary or confinement; one could imagine:

1. The motions piercing and breaking these boundaries (constant)
2. The motions bouncing onto themselves (cycle)
3. All motion and event extinguishing (¿linear?)
4. Constant pressure / friction of the motions against its boundary (constant)
5. A repercussion of scenario 4; deviation of the motions into different directions (new paths). Still the "first" motions continue in friction with the boundary, perhaps yet another new event as a result (¿constant cycle?)

"Image 47" .. "Most left centered close-up of img.[46]"

"Image 48" .. "Most left centered close-up of img.[47]"

…At the situation being described, in order to experience a notion of time, one could picture an observer...

We can describe the image above as en edge of the structure; as for the state of development shown on "Image [45]". The sum of edges enclose a basic cell. From this perimeter the ¿rule? would repeat as well.

...So, coming back to the happenings at an infinite empty space; the experience of said observer would depend on many variables that one may incur, for the interpretation to come; we'll picture one observer at any given point of the structure along its respective perimeter of experience...

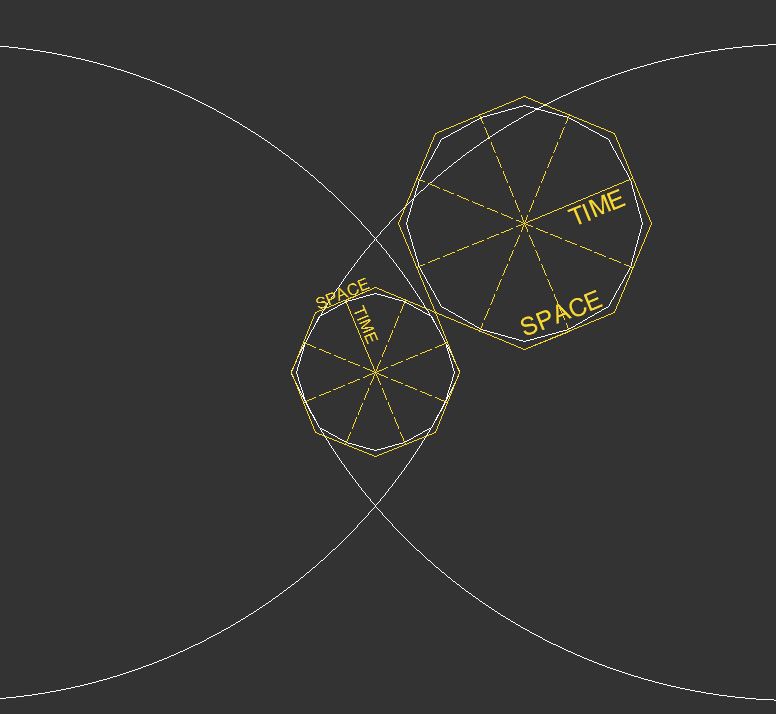
time

Incidence

Some reference to paint a panorama.

• Duality
• Degenerate conics
• Extraordinary conics
• Parallel transport
• Entanglement
• Entropy
• Page Curve
• Unit cell cubic structure
• Hybridization Theory
• Time Dilation
• Banach–Tarski paradox
• Quantum electrodynamics
• Gravitational waves
• General Relativity
• Cavalieri's principle
• Supersymmetry
• Penrose–Hawking Singularity Theorem
• Inflationary theory
• QBism
• Collision detection systems