This is a very nice theorem! Thank you for sharing your clear demonstration of this effect.

As a shy remark: I could not resist making graphical representation of the successively blocked angles.

If we have this simple situation:

ClearAll["Global`*"]

latticePts = Flatten[Table[{nx, ny}, {nx, 10}, {ny, 10}], 1];
radius = .1;
demoxy = {6, 7};
bar[p1_, p2_, llength_] := Rectangle[p1, {llength, Last[p2]}]

(* blocked angle range: *)
\[Phi]range = Module[{\[Phi], \[Delta]\[Phi], dist},
     \[Phi] = ArcTan[#1, #2];
     dist = Norm[{#1, #2}];
     \[Delta]\[Phi] = ArcTan[radius/dist];
     {{dist, \[Phi] - \[Delta]\[Phi]}, {dist, \[Phi] + \[Delta]\[Phi]}}] & @@@ latticePts;

Graphics[{Disk[#, radius] & /@ latticePts, Red, Arrow[{{0, 0}, demoxy}]}, 
 PlotRange -> {{0, Automatic}, {0, Automatic}}, Frame -> True]

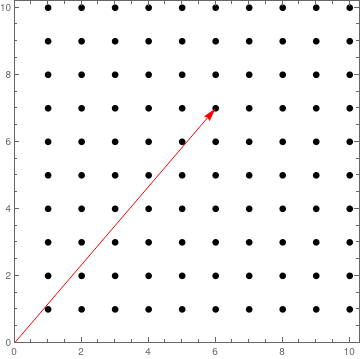
Then the blocked angle ranges look like:

Graphics[{Black, Line /@ \[Phi]range,
  Red, Arrow[{{0, ArcTan @@ demoxy}, {Norm[demoxy], 
     ArcTan @@ demoxy}}],Gray, Opacity[.2], bar[#1, #2, 15] & @@@ \[Phi]range}, 
 AspectRatio -> .8, ImageSize -> 700, Frame -> True, FrameLabel -> {"Norm", "Angle \[Phi]"}]

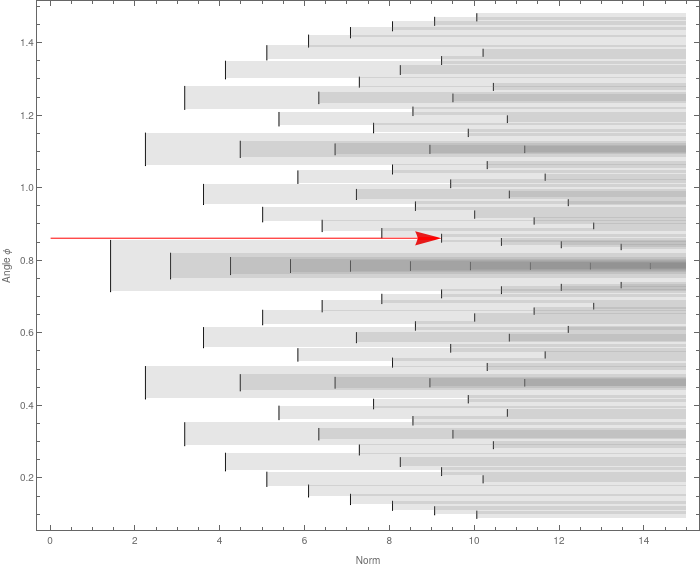
I think this is at least aesthetically pleasing.

I just wanted to add an update based on https://mathworld.wolfram.com/OrchardVisibilityProblem.html

It is assumed that the origin is the point of vision. In fact any light ray from a outside point to the origin lies (symmetrically in the middle) in a visibility strip. Since any visibility strip contains at least a lattice point at its interior, the corresponding tree occlude the light ray for its size exceeds half of this visibility strip.

The importante fact is that the midline is always occluded by a tree, hence the "eye" located at Origin cannot see the light ray ( midline of the strip) that comes from outside.

The eye is a point, it has no extension.