In response to the question and some answers here: https://mathematica.stackexchange.com/q/291951
where we need to efficiently mowing a grass region using the least amount of time/fuel. The region can be concave and/or contain obstacles such as for example:
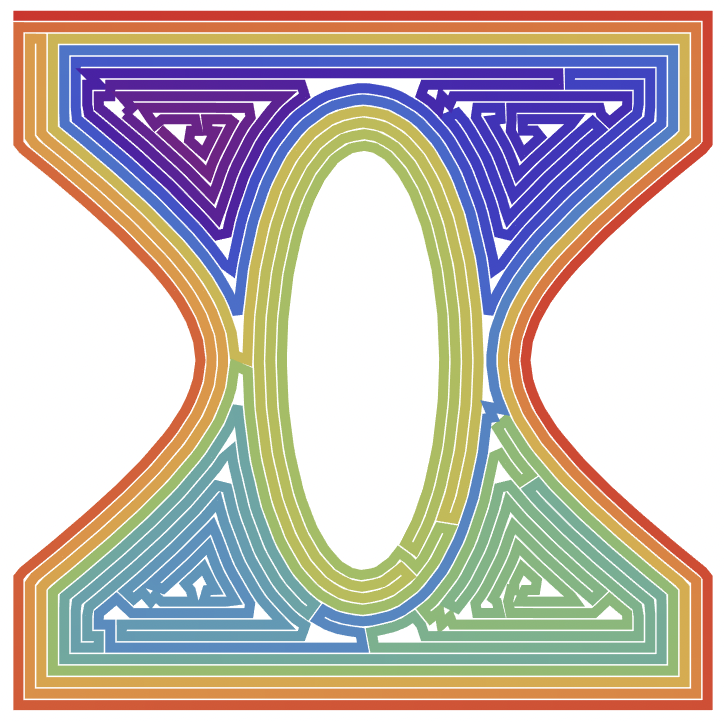
Here's my attempt, which is to take Vitaliy's method but try to mow along isolines of the region as much as possible. Essentially to try to 'spiral' inwards.
eq = x^2/2 - y^2 <= 1 && x^2 + y^2/7 > 1/2 && -3 < x < 3 && -3 < y < 3;
R = ImplicitRegion[eq, {x, y}];
reg = BoundaryDiscretizeRegion[R];
Attempt 1 (times out)
The idea is to discretize the region a certain distance from the boundary, then add 'bridge' edges across the isolines, where these bridges are weighted with a larger penalty. Then call FindShortestTour.
$bds = {};
reg2 = reg;
w = 0.1;
While[reg2 =!= EmptyRegion[2],
(* remesh the curve to have nice sampling *)
AppendTo[$bds, RegionBoundary@BoundaryDiscretizeRegion[
ImplicitRegion[SignedRegionDistance[reg2][{x, y}] <= 0 && -3 < x < 3 && -3 < y < 3, {x, y}], MaxCellMeasure -> {1 -> w}]];
reg2 = BoundaryDiscretizeRegion[RegionErosion[reg2, w]];
]
Length[crossedges = (Join @@ ((Join @@ MapThread[Function[{c1, c2},c1 \[UndirectedEdge] #& /@ c2[[1;;UpTo[1]]]],{#1, Nearest[#2, #1, {All, 1.25w}]}])& @@@ Partition[MeshCoordinates /@ $bds, 2, 1]))]
Output: 2377
Length[cycleedges = UndirectedEdge @@@ MeshPrimitives[UTJoin[$bds], 1][[All, 1]]]
Output: 2683
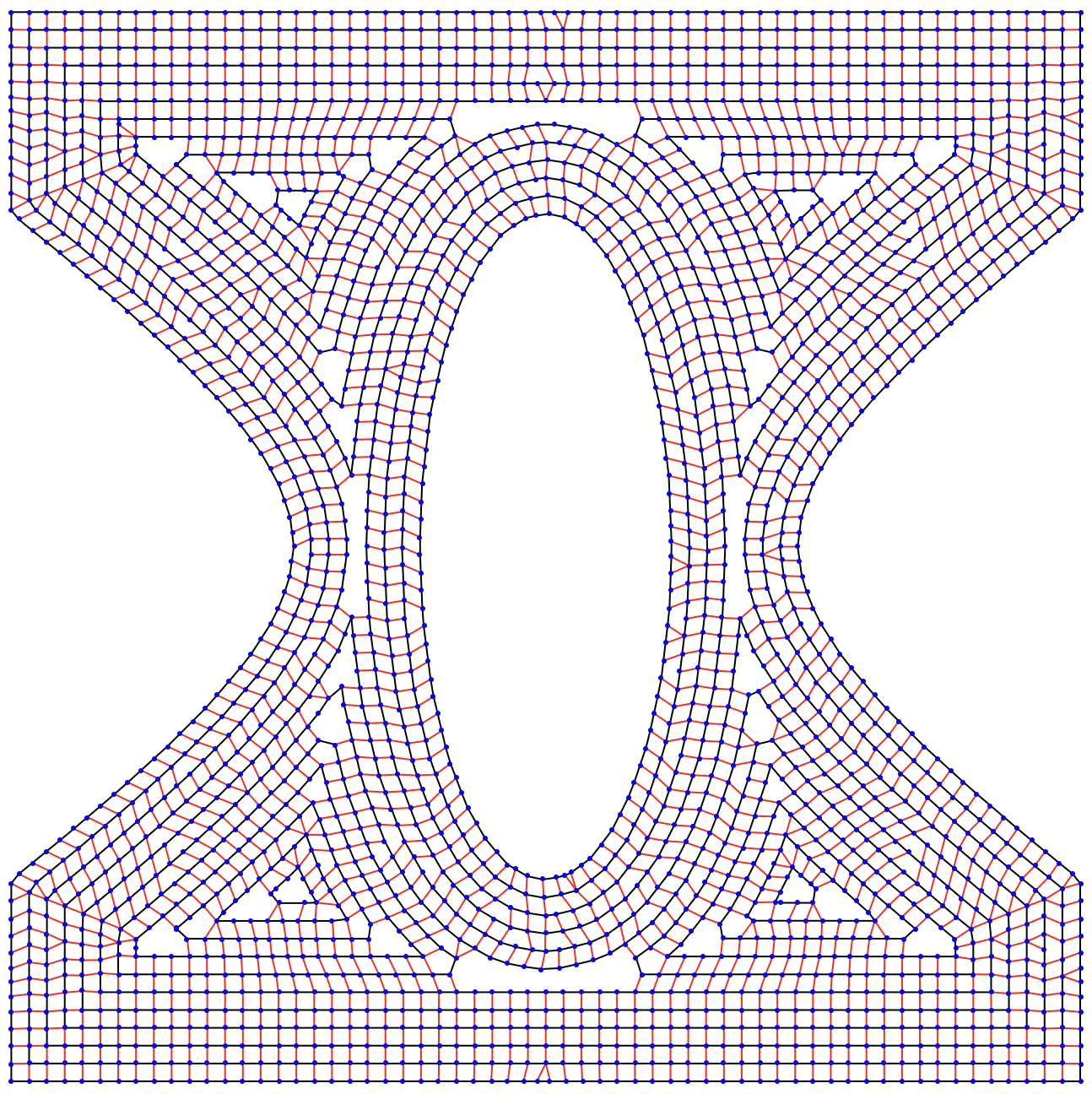
Here we want to travel along the black edges, and will sometimes bridge across the red edges:
Graphics[{
Line[List @@@ cycleedges[[1 ;; -1]]],
{Red, Line[List @@@ crossedges[[1 ;; -1 ;; 1]]]},
{Blue, Point[MeshCoordinates[UTJoin[$bds]]]}
}]
Build the (weighted) graph:
g = Graph[
MeshCoordinates[UTJoin[$bds]],
Join[cycleedges, crossedges],
EdgeWeight ->
Join[(# -> EuclideanDistance @@ #) & /@
cycleedges, (# -> 100 EuclideanDistance @@ #) & /@ crossedges],
VertexCoordinates -> MeshCoordinates[UTJoin[$bds]]
];
But FindShortestTour times out:
FindShortestTour[g]
$Aborted
Attempt 2
To work around this time out, we can pass a point cloud to FindShortestTour instead, lifting into 3D where each isoline will be in its own plane. Jumping 'up' in 3D will be the penalty for changing isolines.
Using $bds from above:
pts = Join @@ MapIndexed[Append[1.25 w (First[#2] - 1)] /@ #1 &, MeshCoordinates /@ $bds];
c = Nearest[pts, {-1000, 1000, 0}][[1]];
tour = FindShortestTour[pts, c, c]; // AbsoluteTiming
Output: {6.93906, Null}
path = pts[[tour[[2]], 1 ;; 2]];
We can visualize the 'spiral' path with a color gradient:
colors = ColorData["Rainbow"] /@ Subdivide[1.0, 0.0, Length[path] - 1]];
Graphics[{AbsoluteThickness[5], Line[path, VertexColors -> colors}]

There's clearly still room for improvement, but it's still a start for sure.