There's a question at math.stackexchange about the smallest 8 cubes to cover a regular tetrahedron. I decided to look at 4 cubes instead.

If a regular tetrahedron has edge length 2, it's not too difficult to find a way to cover it with 4 unit cubes. Can the cubes be smaller?

I took a fourth of the tetrahedron and randomly rotated it a few million times to find the smallest bounding cube. I managed to find a matrix giving a bounding cube with side 0.969975.

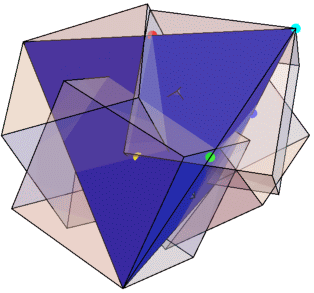
That doesn't look optimal, so I'll ask if anyone can improve the solution with smaller cubes. I give the various steps I took in the attached notebook.

Attachments: