Inspired by the work of the Frisian artist M.C. Escher, I decided to make this little animation:

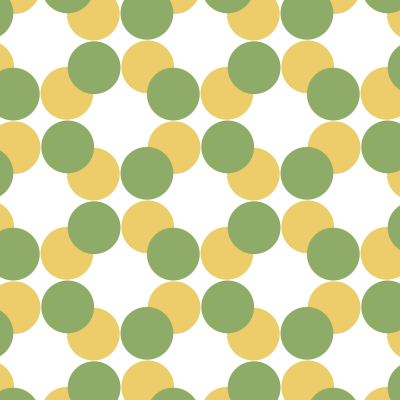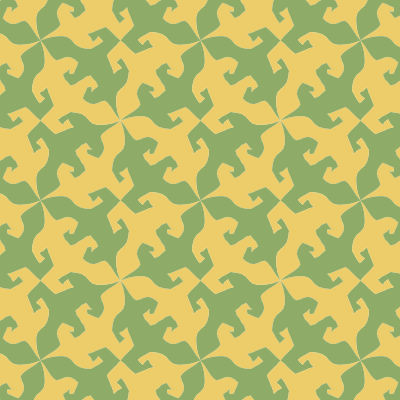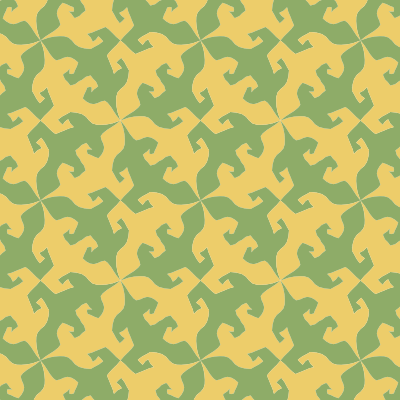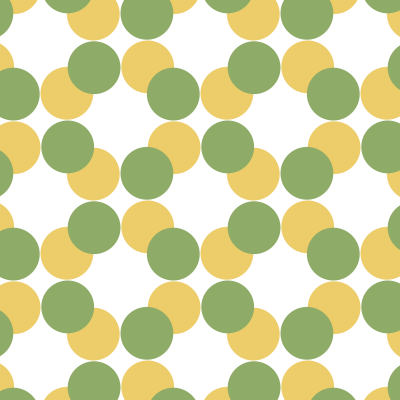
The code is nothing more than linear interpolation between sets of points:

SetDirectory[NotebookDirectory[]];

p1 = {{0.`,0.`},{0.0678`,0.054200000000000005`},{0.1336`,0.09570000000000001`},{0.1831`,0.1257`},{0.2398`,0.1714`},{0.26780000000000004`,0.20850000000000002`},{0.2528`,0.2606`},{0.22760000000000002`,0.3084`},{0.2117`,0.3584`},{0.21930000000000002`,0.41000000000000003`},{0.24550000000000002`,0.4595`},{0.28500000000000003`,0.5056`},{0.34`,0.48260000000000003`},{0.3935`,0.45320000000000005`},{0.4305`,0.43760000000000004`},{0.43820000000000003`,0.39840000000000003`},{0.4303`,0.3698`},{0.3831`,0.3678`},{0.3552`,0.3683`},{0.3925`,0.33180000000000004`},{0.4148`,0.2927`},{0.4339`,0.2671`},{0.49720000000000003`,0.2947`},{0.5356000000000001`,0.33380000000000004`},{0.5789000000000001`,0.3659`},{0.558`,0.4297`},{0.5141`,0.48090000000000005`},{0.5`,0.5`},{0.5`,0.5`},{0.4859`,0.5191`},{0.442`,0.5703`},{0.42110000000000003`,0.6341`},{0.46440000000000003`,0.6662`},{0.5028`,0.7053`},{0.5661`,0.7329`},{0.5852`,0.7073`},{0.6075`,0.6682`},{0.6448`,0.6317`},{0.6169`,0.6322`},{0.5697`,0.6302`},{0.5618000000000001`,0.6016`},{0.5695`,0.5624`},{0.6065`,0.5468000000000001`},{0.66`,0.5174`},{0.7150000000000001`,0.4944`},{0.7545000000000001`,0.5405`},{0.7807000000000001`,0.5900000000000001`},{0.7883`,0.6416000000000001`},{0.7724000000000001`,0.6916`},{0.7472000000000001`,0.7394000000000001`},{0.7322000000000001`,0.7915000000000001`},{0.7602`,0.8286`},{0.8169000000000001`,0.8743000000000001`},{0.8664000000000001`,0.9043`},{0.9322`,0.9458000000000001`},{1.`,1.`}};
p2 = {{1.`,1.`},{1.0396177978506647`,0.8923346254845568`},{1.0553148607198288`,0.8165562085782169`},{1.0612803330660763`,0.7422415758850744`},{1.0593972739777413`,0.6855070651494309`},{1.0410889377634256`,0.6295007686706042`},{0.9985803499841852`,0.5851973901977947`},{0.9483041434655642`,0.5486542747648014`},{0.9100397943346402`,0.495994821587507`},{0.8886126415052703`,0.4220994637695018`},{0.8802712781999131`,0.3410911444732952`},{0.9107680085914569`,0.2922860778674355`},{0.944118750413758`,0.23431580960507237`},{0.9891650545425124`,0.1782359561306078`},{1.0249726000191246`,0.2133888443460414`},{1.0610964405769812`,0.25639761969562114`},{1.0125267563571634`,0.29612575303974287`},{0.9819491132704178`,0.3427388211755879`},{1.042685124568772`,0.3779726220862235`},{1.0923875865214163`,0.4314412022155367`},{1.110695922735732`,0.3701240906516413`},{1.139236037043303`,0.307894872341834`},{1.1672759637805352`,0.24281899830083345`},{1.190159545123539`,0.19293999955865795`},{1.1550875696033072`,0.15058588147025714`},{1.1048113630846865`,0.1033990687684352`},{1.0520783529117537`,0.05681542343084531`},{1.`,0.`}};
rf = RotationTransform[\[Pi]/2, {1, 0}];
p3 = Reverse[rf /@ p2];

colors = {RGBColor[0.9280877328700329, 0.8058790727091572, 0.41541817087124444`],RGBColor[0.5551256603319519, 0.6745729914926235, 0.40725444158653856`]};

ClearAll[GetLines, MakeScene]
GetLines[\[Beta]_] := 
 Module[{\[Alpha], goal1, goal2, goal3, goal, lenp},
  If[0 <= \[Beta] <= 0.5,
   \[Alpha] = 2 \[Beta];

   lenp = Length[p1] + Length[p2] + Length[p3];
   goal = CirclePoints[{0.66, 0.33}, {0.33, 3.97}, lenp];

   {goal1, goal2, goal3} = FoldPairList[TakeDrop, goal, (Length /@ {p3, p2, p1})][[{3, 2, 1}]];
   Polygon[Join @@ {\[Alpha] p1 + (1 - \[Alpha]) Reverse[
         goal1], \[Alpha] p2 + (1 - \[Alpha]) Reverse[
         goal2], \[Alpha] p3 + (1 - \[Alpha]) Reverse[ goal3]}]
   ,
   \[Alpha] = 2 (\[Beta] - 0.5);
   goal1 = Subdivide[0, 1, Length[p1] - 1];
   goal1 = {goal1, goal1}\[Transpose];

   goal2 = Subdivide[1, 0, Length[p2] - 1];
   goal2 = Thread[{1, goal2}];

   goal3 = Subdivide[1, 0, Length[p3] - 1];
   goal3 = Thread[{goal3, 0}];
   Polygon[Join @@ {(1 - \[Alpha]) p1 + \[Alpha] goal1, (1 - \[Alpha]) p2 + \
\[Alpha] goal2, (1 - \[Alpha]) p3 + \[Alpha] goal3}]
   ]
  ]
MakeScene[\[Alpha]_] := Module[{in, shape},
  in = GetLines[\[Alpha]];
  shape = {in, Rotate[in, \[Pi], {0.5, 0.5}]};
  shape = Riffle[colors, shape];
  shape = Rotate[shape, #, {0, 0}] & /@ Range[0, 3 \[Pi]/2, \[Pi]/2];
  shape = Translate[shape, Tuples[{-2, 0, 2}, 2]];
  shape
  ]

To animate it using manipulate use:

Manipulate[Graphics[MakeScene[\[Tau]], PlotRange -> 2.5], {\[Tau], 0, 1}]

And to output the animation I used:

n=150;
ClearAll[Nonlineartime]
Nonlineartime[t_]:=0.5LogisticSigmoid[25(t-0.2)]+0.5LogisticSigmoid[25(t-0.75)]
Plot[Nonlineartime[t],{t,0,1}]
ts=Table[Nonlineartime[t],{t,Subdivide[0.0,1,n]}];
ts[[{1,-1}]]={0.0,1.0};
imgs=Table[Rasterize[Graphics[MakeScene[t],PlotRange->2.5,ImageSize->400],"Image"],{t,ts}];
Export["geckotransform.gif",imgs~Join~Reverse[imgs],"DisplayDurations"->0.03]