Move Along

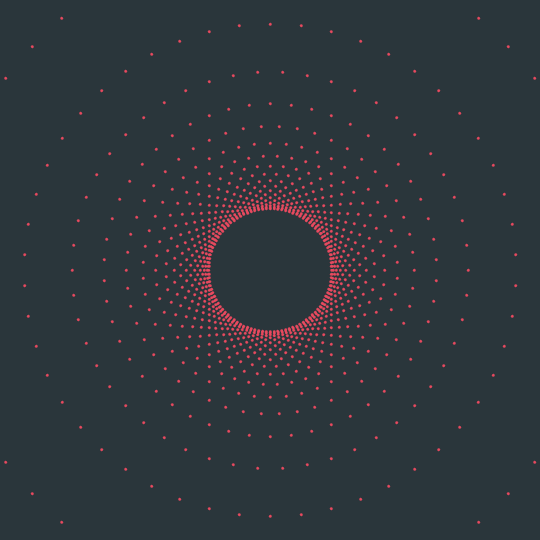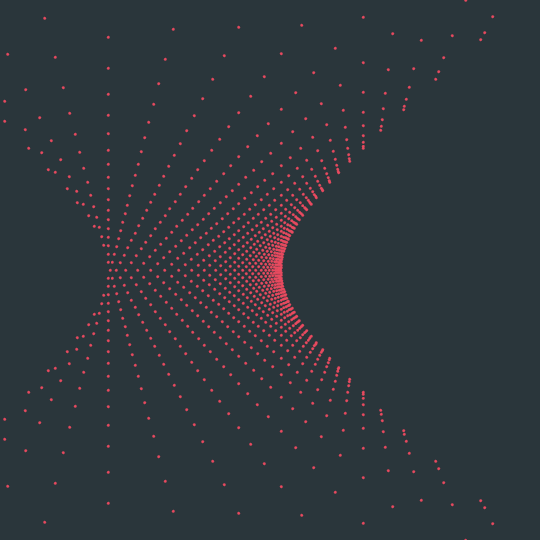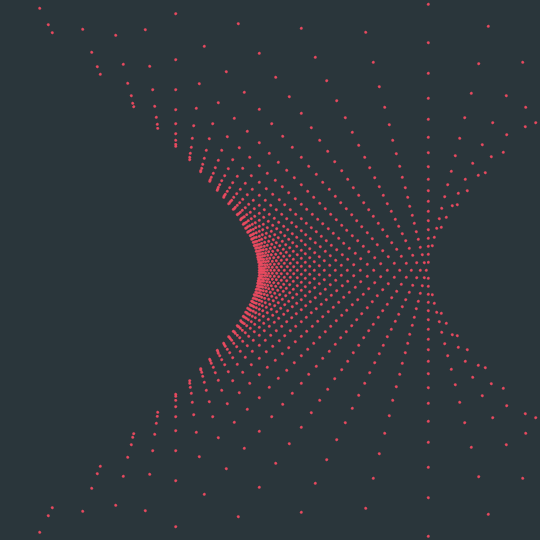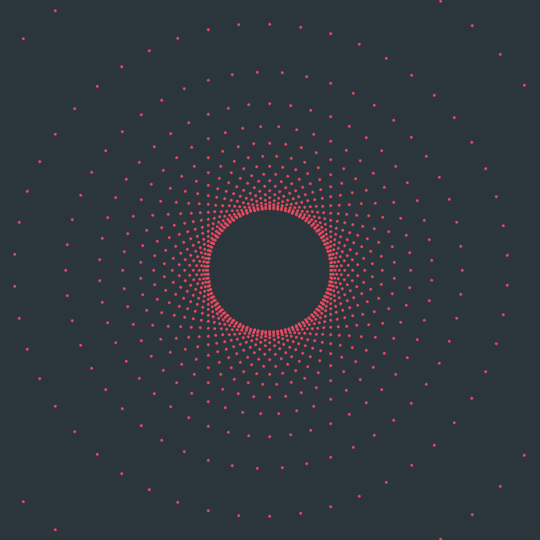
Consider a circle of latitude at height $z=-3/4$ on the unit sphere. The orthogonal complements of unit vectors on the circle are planes through the origin in $\mathbb{R}^3$, which we can also interpret as lines in $\mathbb{RP}^2$, the real projective plane. In order to visualize these lines, take the intersection of the planes with the plane $z=1$. These lines will form the envelope of a circle in the $z=1$ plane.

Now, as we rotate the unit sphere around the $y$-axis, the lines in the $z=1$ plane will form the envelopes of a family of conics. What the animation actually shows are the points of intersections of the lines corresponding to 50 equally-spaced points on this rotating circle on the unit sphere.

Here's the code:

DynamicModule[{m, p, a, \[Theta] = 0., t = \[Pi]/1., r = -0.75, lines,
   linepairs, dots, cols = RGBColor /@ {"#E84A5F", "#2A363B"}},
 Manipulate[
  m = RotationMatrix[t, {Cos[\[Theta]] Sin[\[Phi]], Sin[\[Theta]] Sin[\[Phi]], Cos[\[Phi]]}];
  p[s_] = m.{Sqrt[1 - r^2] Cos[s], Sqrt[1 - r^2] Sin[s], r};
  a = m.{0, 0, 1};
  lines = 
   Table[InfiniteLine[{{-p[s][[3]]/p[s][[1]], 
       0}, {0, -p[s][[3]]/p[s][[2]]}}], {s, 0, 2 \[Pi] - 2 \[Pi]/50, 2 \[Pi]/50}];
  linepairs = Subsets[lines, {2}];
  dots = DeleteCases[RegionIntersection @@ # & /@ linepairs, 
    EmptyRegion[2]];
  Graphics[{White, PointSize[.005], cols[[1]], dots}, 
   ImageSize -> 540, Frame -> False, Background -> cols[[-1]], 
   PlotRange -> 5], {\[Phi], 0., \[Pi]/2}]
 ]