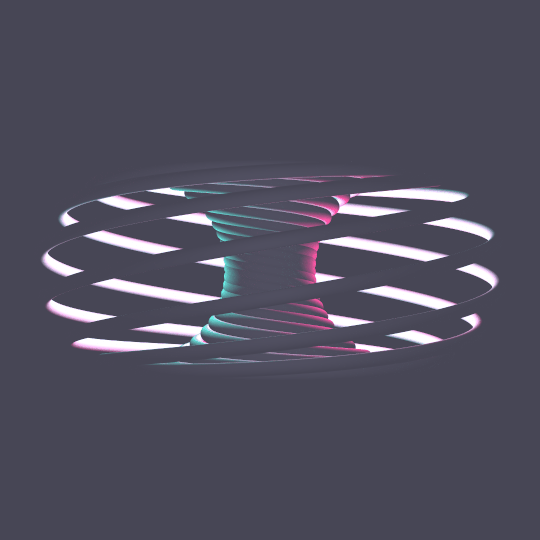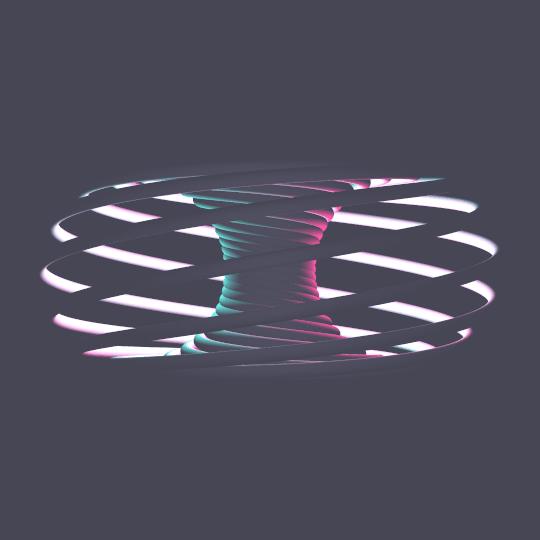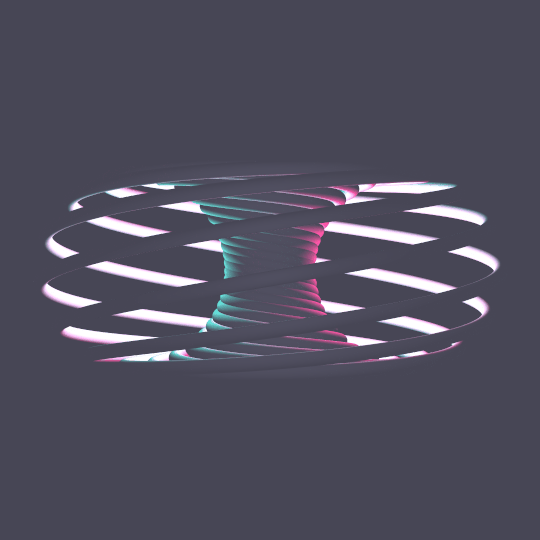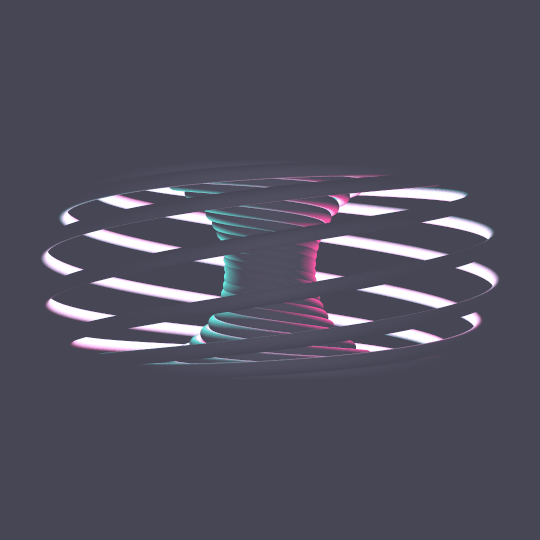
Rise Up
Continuing the torus knot theme (1, 2, 3). This is just a simple rotation of a $(29,5)$-torus knot. It's entirely three-dimensional, but because it's much simpler to parametrize torus knots on the Clifford torus in 4D, I am as usual parametrizing there and then stereographically projecting to 3D.
Here's the code:
Stereo3D[{x1_, y1_, x2_, y2_}] := {x1/(1 - y2), y1/(1 - y2), x2/(1 - y2)};
pqtorus[t_, ϕ_, p_, q_] := 1/Sqrt[2] {E^(p I (t + ϕ/p)), E^(q I t)};
With[{viewpoint = {0, 3, 0}, n = 450*29, p = 29, q = 5,
cols = RGBColor /@ {"#F21368", "#22C7A9", "#474655"}},
Manipulate[
Graphics3D[
{Tube[Table[Stereo3D[Flatten[ReIm /@ pqtorus[t, -ϕ, p, -q]]], {t, 0., 2 Pi, 2 Pi/n}], .07]},
PlotRange -> 2.7, ViewPoint -> viewpoint, ViewAngle -> Pi/9,
ViewVertical -> {0, 0, -1}, Boxed -> False,
Background -> cols[[-1]], ImageSize -> 540,
Lighting -> {{"Point", cols[[1]], {3/4, 0, 0}}, {"Point", cols[[2]], {-3/4, 0, 0}},
{"Ambient", cols[[-1]], viewpoint}, {"Point", Darker[cols[[-1]], .87], viewpoint}}],
{ϕ, 0, 2 Pi/q}]
]