I have more or less found the solution to this: plotting variables in pairs with the correct choice of maximum and minimum values and overlapping them.

The output is:

Once checked that this visual representation covers the whole region, it is now possible to identify the curves that define the edges and draw only them.

I guess the way to go is trying to get a discrete representation as a cloud of points, I was hoping to be able to get a complete region.

Going back to the ParametricPlot output, what do the different shades of blue mean?

EDIT:

The dark blue region is the place where different combinations of parameter values give the same (X,Y) combination. Maybe this superposition of points is the reason why ParametricRegion is not able to give an output.

I have tried to do it in two different ways.

1. Using ParametricRegion:

R = ParametricRegion[{Xt[X2, Th3, La1, La2, La3, La4, La5] /. {X2 -> X2in, La3 -> La3in} /. {Th3 -> Th3in, La4 -> La4in, La5 -> La5in}, Yt[X2, Th3, La1, La2, La3, La4, La5] /. {X2 -> X2in, La3 -> La3in} /. {Th3 -> Th3in, La4 -> La4in, La5 -> La5in}}, {{La1, dA1min, dA1max}, {La2, dA2min, dA2max}}];

And trying to represent it by:

Region[R]

This is not able to give a result (it is still running after about 10h and 8GB of RAM).

2. Using ParametricPlot

ParametricPlot[{{Xt[X2, Th3, La1, La2, La3, La4, La5], Yt[X2, Th3, La1, La2, La3, La4, La5]}} /. {X2 -> X2min, Th3 -> Th3min, La3 -> dA3min, La4 -> dA4min, La5 -> dA5min}, {La1, dA1min, dA1max}, {La2, dA2min, dA2max}, AspectRatio -> Automatic]

This is able to give a result relatively fast (40s and <2GB of RAM).

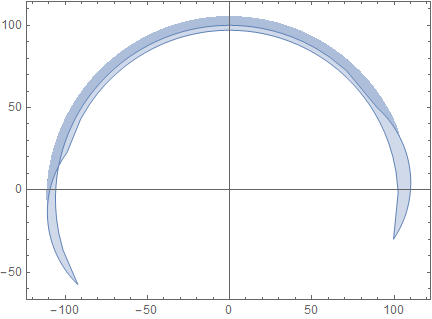
But unfortunately, ParametricPlot can't plot regions depending on more than 2 variables.

Just to give some insight into the problem, (Xt, Yt) give the position of a point from a body in a machine. I need to obtain the 'range of action' of this point. I need to find the contour of the area (or the area itself) in which the point can be located. The problem is that the point depends on 5 (6) variables.

It is suggested that you post any code you have already written so your problem can be examined more closely.