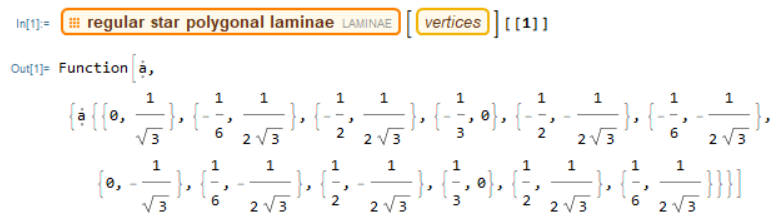
Beautiful! Slightly modify the code above we can have the following
anim = With[{\[Delta] = 1/12,
cols = RGBColor /@ {"#07090e", "#2bb3c0", "#faf7f2"}},
Table[Graphics[Reverse[Table[s = Mod[r + i, 3/2];
{Blend[cols, LogisticSigmoid[8 (s - 1/2)]],
Polygon@Map[RotationTransform[s], star52[2*s], {2}]}, {i, 0,
3/2 - #, #}]] &[\[Delta]], PlotRange -> 1,
ImageSize -> 540, Background -> cols[[-1]]], {r, 0, \[Delta],
0.003}]];
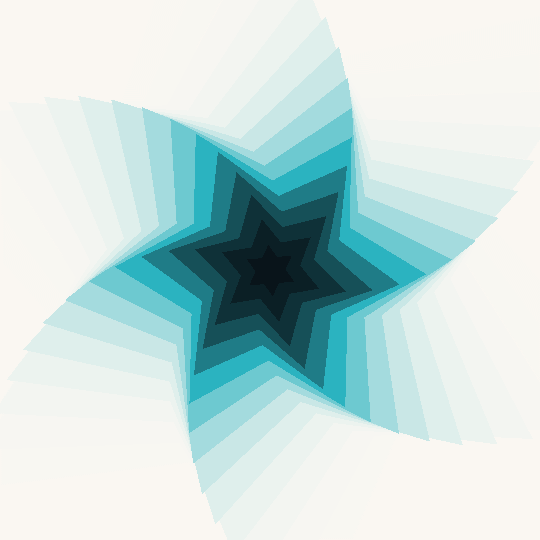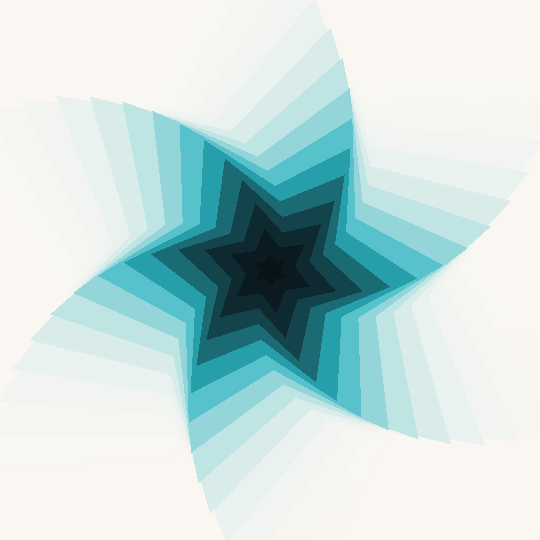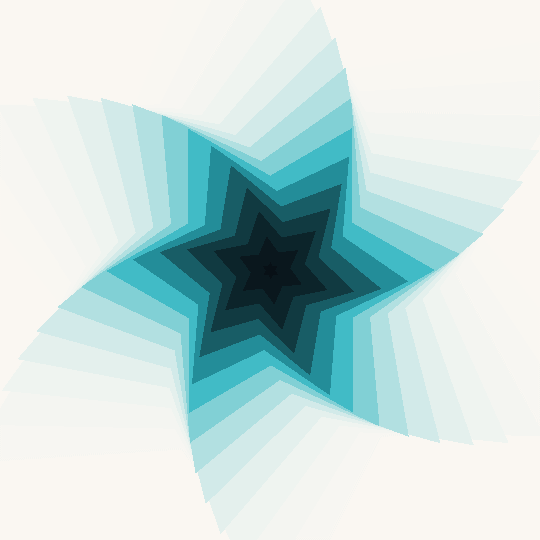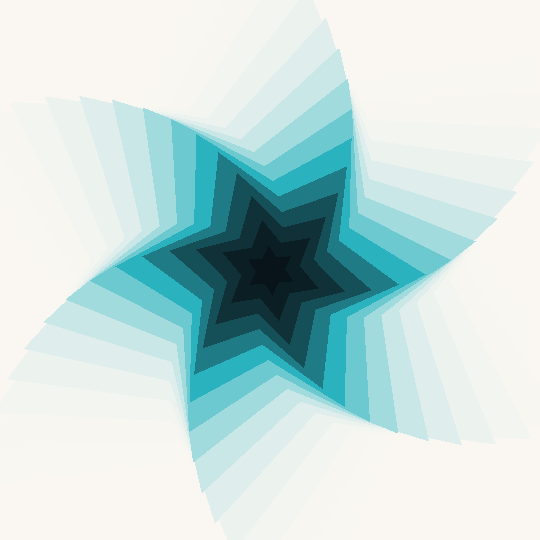
Animate the plot:
ListAnimate[anim~Join~anim]
where star52 is a function from
EntityClass["Lamina", "RegularPolygram"][EntityProperty["Lamina", "Vertices"]][[1]]