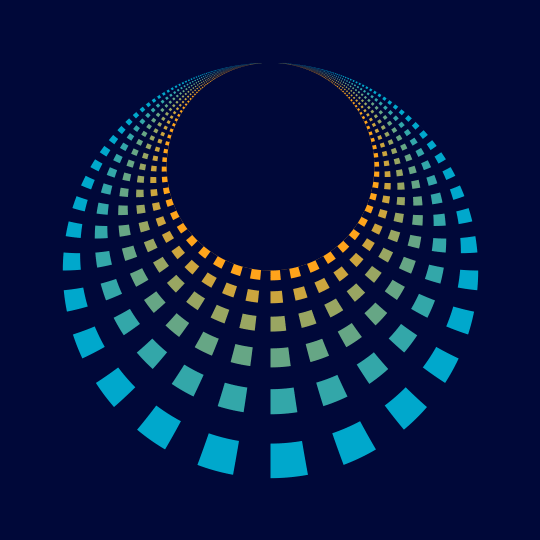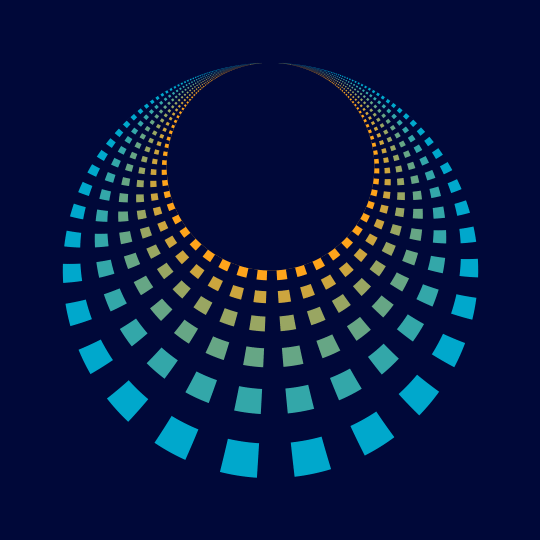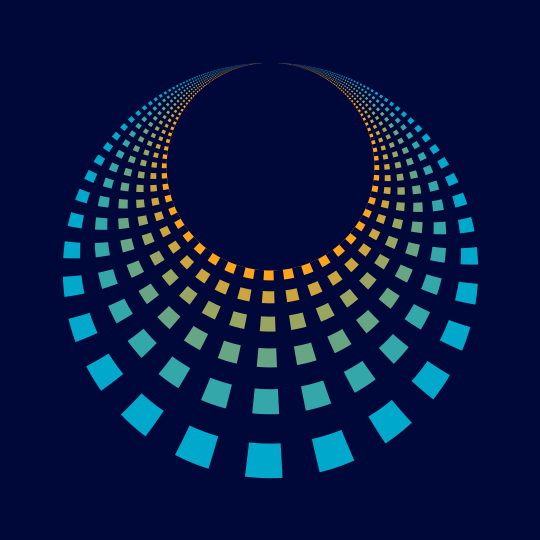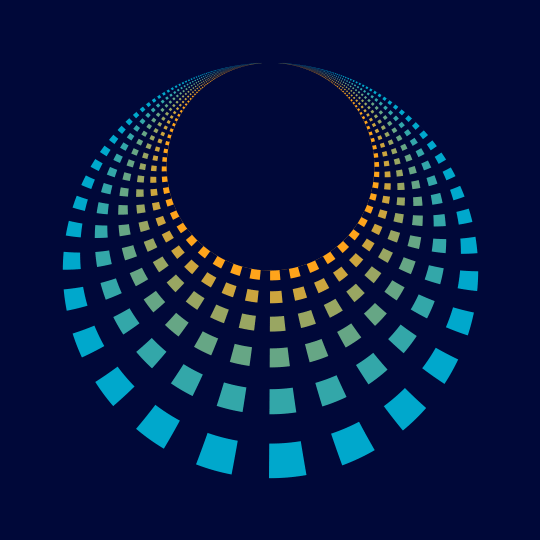
Vanishing Point
This is essentially the same setup as :eyes:: the map $f(z) = \frac{-4i}{z}$ maps the infinite strip $\{z \in \mathbb{C} : 1 \leq \operatorname{Re}(z) \leq 2\}$ to the region between the two circles $\{z \in \mathbb{C}: |z+i| = 1\}$ and $\{z \in \mathbb{C}: |z+2i|=2\}$, so the square grid in the strip gets mapped to a right-angled grid in the region between circles.
Code for a (very slow) Manipulate (see this comment for the process of exporting to GIF):
With[{r = 20, d = 11,
cols = RGBColor /@ {"#00a8cc", "#ffa41b", "#000839"}},
Manipulate[
Graphics[{EdgeForm[None],
Table[{Blend[cols[[;; 2]], (i - 1)/(Length[#] - 1)],
Polygon[Join[#[[i, 1]], Reverse[#[[i, 2]]]]]},
{i, 1, Length[#]}]
&@Partition[Table[ReIm[(-4 I)/(x + I t)], {x, 1., 2, 1/d}, {t, -50, 50, .01}], 2],
cols[[-1]],
Polygon[Join[#[[1]], Reverse[#[[2]]]]] & /@
Partition[Table[ReIm[(-4 I)/(1 + t + I (y - u))], {y, Join[Table[s, {s, -r, r, 1./d}]]}, {t, -.01, 1, .01}], 2]},
ImageSize -> 540, PlotRange -> {{-2.6, 2.6}, {-4.6, .6}},
Axes -> None, Background -> cols[[-1]]],
{u, 0, 2/d}]
]