Thank you for your input. I realize that I may have explained myself poorly. I am not arguing that no multiway system has an arrow of time, of course. Your example is clear in this regard, it features an obvious arrow of time, I am not arguing against that.

I am saying that there is a class of systems, that I called "complete multiway systems" or "multiway systems with a complete set of rules", for which after a few steps the arrow of time necessarily ceases to exist.

I am also claiming that a universe where every possible rule can be applied (such as the one referred to by Wolfram) is a complete multiway system, and therefore it may not have an arrow of time and may not describe our own universe.

While writing this, I realized that in my previous post I defined a "complete set of rules", but I didn't give a definition of "complete multiway system". I thought that it could be inferred from the context, but this may instead be a source of misunderstanding. I will define the concept here:

definition: complete multiway system

Consider a multiway system that evolves from some initial conditions through the application of a set of rules $\mathcal{R}$. Let $C$ be the set of all possible hypergraphs that are reached by the multiway system during its evolution. The multiway system is complete iff $\mathcal{R} = \mathcal{R}_C$, i.e. if the set of rules used is a complete set of rules of $C$.

In other words, the rules must be able to go from every element in $C$ to every other element, and at the same time, the application of a rule to an element of $C$ always returns another element of $C$.

A couple of examples made with string substitution systems:

• The set of rules $\mathcal{R} = \{a \rightarrow b, b \rightarrow a\}$ is a complete set of rules of $C = \{a,b\}$. Evolving from the initial condition $\{a\}$ generates a complete multiway system.

• The set of rules $\mathcal{R} = \{a \rightarrow b, b \rightarrow a, a \rightarrow c\}$ is still a complete set of rules of $C = \{a,b\}$. But this time, evolving from the initial condition $a$ leads to the state $c \notin C $ and therefore the multiway system is not complete.

Some less trivial examples:

• Consider set of integer numbers $\mathbb{Z}$ coupled with the set of rules $\{\text{add}_i \forall i \in \mathbb{Z}\}$ that add an integer number, i.e. $\text{add}_i: n \rightarrow n+i$. Starting from some integer number as initial condition you obtain a complete multiway system.

• The set of all possible planar graphs, coupled with all the possible rules that preserve planarity. This too generates complete multiway systems.

I hope it is now clear why your example is not a complete multiway system and therefore is not subject to the theorem I proved in the previous post.

I hope it is also clear why the system with all possible rules of which Wolfram speaks is indeed a complete multiway system and therefore the theorem applies.

This discussion with you is surely very stimulating, you have found something I hadn't considered, and the prospects are very exciting.
I thought about your counterexample, but I don't think it is a complete system, because the rules given are able to go from (1,1) to (1,2) and (1,1) (as you pointed out), but if you start from (1,2), you can only go to (1,1). This means that there is no rule able to link (1,2) to itself. Adding this rule manually would freeze the system.

Anyway even if this examples may not be perfect, I can finally see your point. The next counterexample you would probably give would be something like the following:

Rules:
{{1, 2}, {3, 2}} -> {{1, 2}, {3, 2}},
{{1, 2}, {3, 2}} -> {{1, 2}, {2, 3}},
{{1, 2}, {2, 3}} -> {{1, 2}, {2, 3}},
{{1, 2}, {2, 3}} -> {{1, 2}, {3, 2}}

Initial Condition: {{{1, 2}, {3, 2}}}

Which should generate a multiway graph like this:

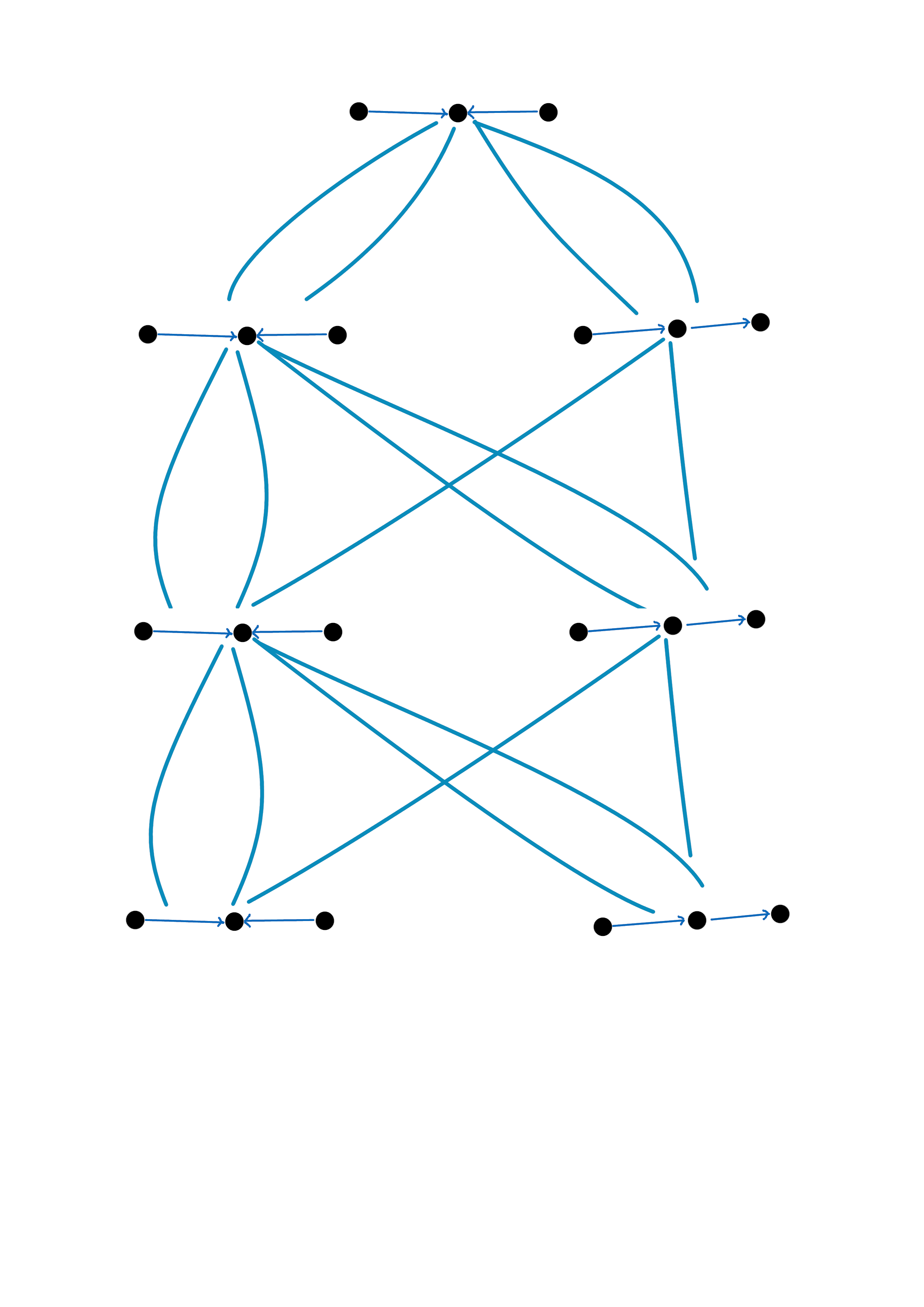
Since there are two ways to apply the rule {{1, 2}, {3, 2}} -> {{1, 2}, {2, 3}} to the state {{1, 2}, {3, 2}}, I have drawn two links. Now, the issue is subtle, because the two links technically represent the same update event. There are then two possible interpretations:

• It may be that the double link is just redundant information. The physics of the system is described by the fact that there is an update event and it doesn't matter how many redundant links represent the event. If this interpretation is correct, the system is frozen, the theorem holds, and I am confident that it holds in every case.

• If instead the double link carries a physical meaning (something like doubling the probability of the event), then you are right. Applying every possible rule at the same time does generate a system with an arrow of time, and all the relevant information is encoded in the number of ways a rule can be applied to go from a state to another. (This gives me a nice idea, but it deserves another post)

The only way to decide between these two options is to know how to calculate path weights in the wolfram model in order to reproduce quantum mechanics. I think that Jonathan Gorard has worked on the issue, but honestly I couldn't understand what solution he may have found.

If you can tell me more about it, I would really appreciate it.

Actually I now get your point of there being two ways to apply one rules. That's right.