In the "Neat Examples" section of documentation for ToElementMesh there is another way to do this. Follow the LINK.

Here's a related challenge: how can I extract the line segments in this image as Line objects?

Show[PolyhedronData["Spikey"], Boxed -> False, Lighting -> {White}]

This is very cool, thanks for sharing!

Here is a variation to transform a (black and white) image to a graphics expression, while taking into account multiple regions.

Throughout this post I will use the following test image:

image = Import["c:\\Users\\arnoudb.WRI\\paw.png"]

This is a helper function which uses MorphologicalComponents to split the image up into a list of images, each containing a single coherent part of the original image:

ImageToParts[image_] := Module[{components, max},
 components = MorphologicalComponents[Binarize@ColorNegate[image]];
 max = Max[Union[Flatten[components]]];
 parts = Table[Image[components /. Table[i -> If[i == j, 1, 0], {i, max}]], {j, max}]
]

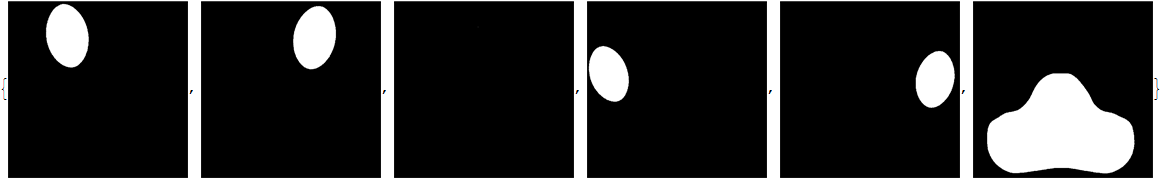
And here is an example of what it does (the third image is a tiny speck in the original):

ImageToParts[image]

This function uses the helper function to split up the original image and find the 'shortest tour' for the edge points of each sub-image:

ImageToGraphics[image_] := Module[
 {dims, parts, edges, points, shortest, smooth, prims},
 dims = ImageDimensions[image];
 parts = ImageToParts[image];
 prims = Table[
  edges = EdgeDetect[part];
  points = {Last[#], 1+Last[dims] - First[#]} & /@ N@Position[ImageData[edges], 1];
  shortest = FindShortestTour[points][[2]];
  smooth = Mean /@ Partition[points[[shortest]], 8, 1, {-1, 1}];
  Polygon[smooth],
  {part, parts}];
 Graphics[
 {EdgeForm[Black], Opacity[.5], Red, prims},
 ImageSize -> dims,
 PlotRange -> {{1, First[#]}, {1, Last[#]}} &[dims]
 ]
]

And here is the result, when calling it on the test image:

graphics = ImageToGraphics[image]

And this shows how the test image and the graphics expression match:

Overlay[{image, graphics}]