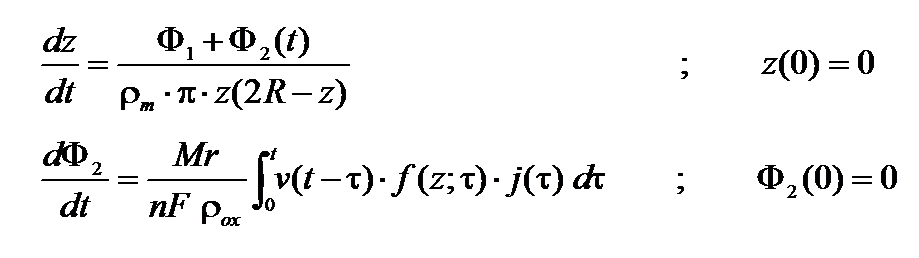Hi everyone
I'm trying to solve a particular physical problem that leads to a system of integro-differential equation that never I found neither solved. The system is described by two state variables z(t) and $\Phi_2(t)$ ; $\Phi_1$ , $\rho$, $\pi$, Mr, nF and R are known constants. In the convolution integral, the functions v(t-$\tau$) and f(z) are known; the last one is coupled with the solution of the first differential equation. The function j( $\tau$) is also a solution of other differential equation or can be written as linear combination of known functions.
I would like use the native functions of Mathematica to solve this system before to try an Euler or collocation method. If someone has experience in this kind of problems I would appreciate any suggestion and comments.
Regards Javier Navarro