Here another version using Play. Imagine you got an analytic function:

f[t_] := Sin[440 2 Pi t Sin[10 t]]

It is easy to play it:

Play[f[t], {t, 0, 1}]

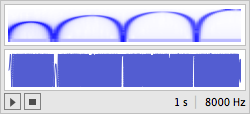
Now if you do not have function but just irregularly sampled data:

data = {#, f[#]} & /@ {0}~Join~RandomReal[1, 10000]~Join~1;
Short[data]
(*{{0,0},{0.366519495348991`,0.749201172621884`}, ....,{0.19676826435559214`,-0.8154545405493382`}}*)

you can recreate sound closely using Interpolation given that sampling is detailed:

g = Interpolation[data, InterpolationOrder -> 0];
Play[g[t], {t, 0, 1}]

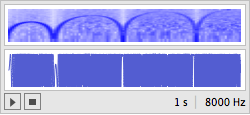
Note sound is different but close enough.