We can calculate the coverage (of the sun) for each of your photo. And inside the photo we can also find the time when it is taken. Using those 2 pieces of information we should be able to identify the location(s?) of your photo right? I.e. not using GPS, but using the coverage, and the times taken...

Wouldn't it be cool if you could find your location just from the photos? I think this would be possible right? If you know the time and the coverage (image analysis), it should be possible right?

Interesting idea. I wish I would know enough celestial mechanics to compute something like this.

It's quite tricky, the coverage is the easy bit, but i need the moon-earth and sun-earth distance at the time around the event... It should be in the PlanetaryMoonData / StarData, but I can't get it to work... You'll hear from me...

Hi Vitaliy,

In general: YES. But with multiple photos you can guess. Let me explain:

The path of the total solar eclipse can be obtained by:

GeoGraphics[{GeoStyling[None], 
  SolarEclipse[DateObject[{2017, 1, 1, 0, 0}], "GraphicsData", 
   EclipseType -> "Total"]}, GeoProjection -> "Equirectangular", 
 GeoRange -> "World"]

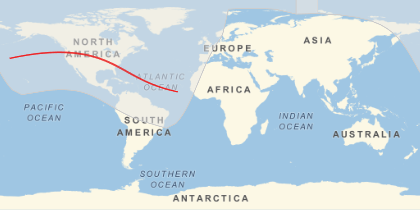
Now the red line is where there is total eclipse. So for any given moment in time it is a 'point'. Now 90% coverage (at the same moment) will be seen by all the people a 'circle' around that point, and the same for 80%, 70% ....10%....

So for any given moment in time during the eclipse you will have people with 100%, and the further you go away from that point the coverage decreases.

Now from your photos we have different times, and different coverages. So for each time we can find the center of the eclipses, and plot those circles with the different coverages. Where these intersect, there should be your location.

If I knew the exact orientation of your camera I would only need 1 photo.

It is by no means an easy calculation, but we can do many simplifications and maybe somethings comes out of it.

Cheers,

Sander!

Indeed, the line is the central path. And the polygon is the area with 100% coverage. If you go outside that you will 90%, 80%, 70%. But note that these polygons is not for a single time, it is the 'sum' for the entire event. For a specific moment the 100% coverage will be something like a disk, and 90% a slightly larger disk, et cetera....

I'm pretty sure Wolfram has these algorithms in house to calculate these polygons. But, as of know, I don't think we have access to them...

Thanks Vitaliy, let me try some magic ;-)