WOLFRAM MATERIALS for the ARTICLE:
Cantarella, J., Deguchi, T. and Shonkwiler, C. (2014),
Probability Theory of Random Polygons from the Quaternionic Viewpoint.
Commun. Pur. Appl. Math., 67: 1658-1699.
https://doi.org/10.1002/cpa.21480
https://arxiv.org/abs/1206.3161v2
Updated figure (see discussion below):
Original figure:
Article abstract
In 1997, Jean-Claude Hausmann and Allen Knutson introduced a natural and beautiful correspondence between planar n-gons and the Grassmann manifold of 2-planes in real n-space. This construction leads to a natural probability distribution and a natural metric on polygons which has been used in shape classification and computer vision. In this paper, we provide an accessible introduction to this circle of ideas by explaining the Grassmannian geometry of triangles. We use this to find the probability that a random triangle is obtuse, which was a question raised by Lewis Carroll. We then explore the Grassmannian geometry of planar quadrilaterals, providing an answer to Sylvester’s four-point problem, and describing explicitly the moduli space of unordered quadrilaterals.
Isometries
In our paper "Probability theory of random polygons from the quaternionic viewpoint", Jason Cantarella, Tetsuo Deguchi, and I showed how to put a highly-symmetric measure on the space of (planar or space) $n$-gons using a connection between polygon spaces and Stiefel and Grassmann manifolds first described by Hausmann and Knutson. For example, there is a (generically) $2^n$-to-1 map from the Stiefel manifold $V_2(\mathbb{R}^n)$ of 2-frames in $\mathbb{R}^n$ (i.e., $n \times 2$ matrices with orthonormal columns) to the moduli space of $n$-gons in the plane of perimeter 2 up to translations.
The important upshot from our perspective is that the Stiefel manifold has a natural (left) $O(n)$ action and corresponding invariant Riemannian metric and probability measure (namely, Haar measure). This means that $O(n)$ acts transitively on the space of planar $n$-gons (which is somewhat surprising) and that it's straightforward to put an $O(n)$-invariant Riemannian metric on the space of $n$-gons.
In particular, $SO(3)$ acts by isometries on the space of triangles. The GIF shows the orbit of the equilateral triangle under the action of the one-parameter subgroup of rotations around the axis $(-1/2,1/2,-1/\sqrt{2})$.
Here's the code (which I don't explain, but which is hopefully not too opaque; to see why the FrameToEdges map is magically the right thing, see one of the aforelinked papers or these slides):
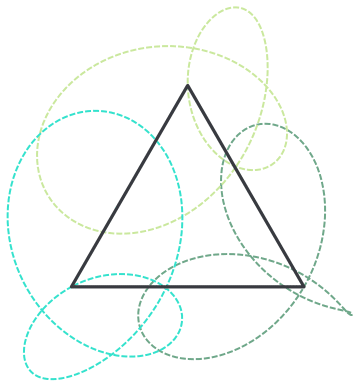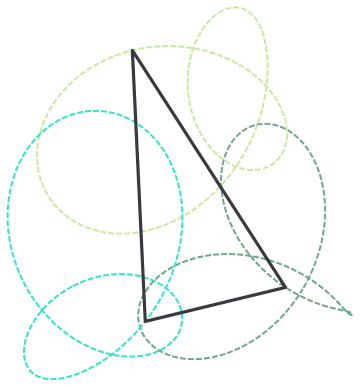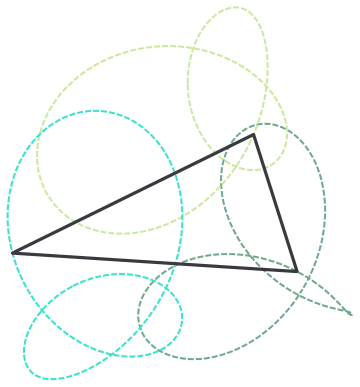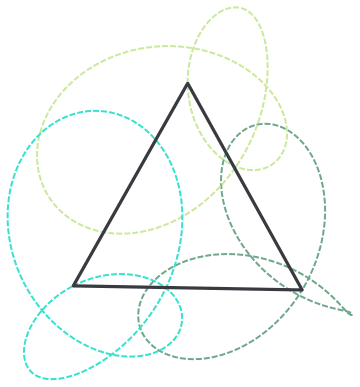
ToComplex[{x_, y_}] := x + I y;
ToReal[z_] := {Re[z], Im[z]};
FrameToEdges[frame_] := ToReal[ToComplex[#]^2] & /@ Transpose[frame];
FrameToVertices[frame_] := Accumulate[FrameToEdges[frame]];
Manipulate[Module[{mat, frame, rotatedframe, verts, com},
mat = RotationMatrix[
t, {Cos[\[Theta]] Sin[\[Phi]], Sin[\[Theta]] Sin[\[Phi]],
Cos[\[Phi]]}] /. {\[Theta] -> 3 \[Pi]/4, \[Phi] -> 3 \[Pi]/4};
frame =
Transpose[
ToReal[Sqrt[#]] & /@ (E^(I #)*2/3 & /@
Range[0, 4 \[Pi]/3, 2 \[Pi]/3])];
rotatedframe = frame.Transpose[mat];
verts = FrameToVertices[rotatedframe];
com = Mean[verts];
verts = RotationMatrix[t].# & /@ (# - com & /@ verts);
Graphics[{FaceForm[None],
EdgeForm[
Directive[RGBColor["#383a40"], Thickness[.009],
JoinForm["Round"]]], Polygon[verts]}, PlotRange -> .8,
ImageSize -> 540, Background -> RGBColor["#F9F9F9"]]],
{t, 0, 2 \[Pi]}]
