You might also like this:

Manipulate[
 VectorPlot[{u (1 - u + (-1 + E^(-u \[Alpha]1)) v),  v (\[Beta]1 - E^(-u \[Alpha]1) \[Beta]1 - \[CapitalGamma]1)}, {v, -5, 
   5}, {u, -5, 5}, StreamPoints -> 40, Epilog -> {Red, PointSize[0.02], Point[Evaluate[
      Select[{v, u} /. (sols[[1 ;; 3]] /. {\[Alpha] -> \[Alpha]1, \[Beta] ->  \[Beta]1, \[CapitalGamma] -> \[CapitalGamma]1}), !MemberQ[#, Undefined] &]]]}], {{\[Alpha]1, 0.795}, 0, 2}, {{\[Beta]1, 1.79}, 0, 2}, {{\[CapitalGamma]1, 1.315}, 0, 3}]

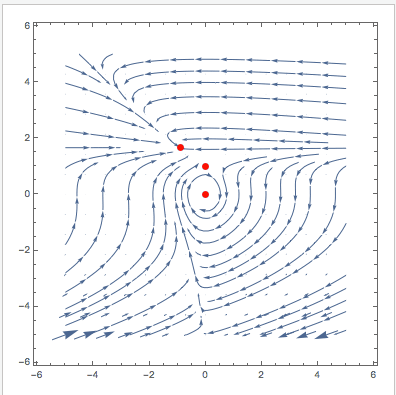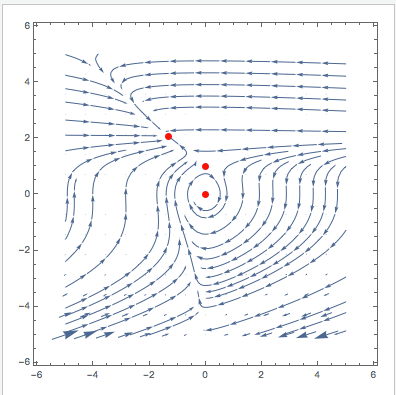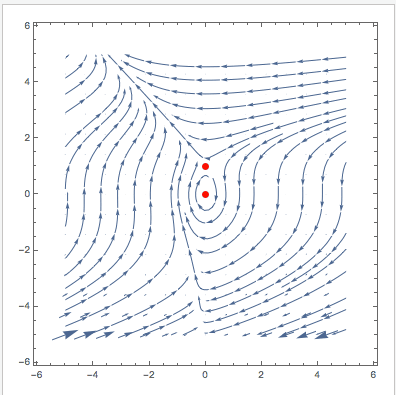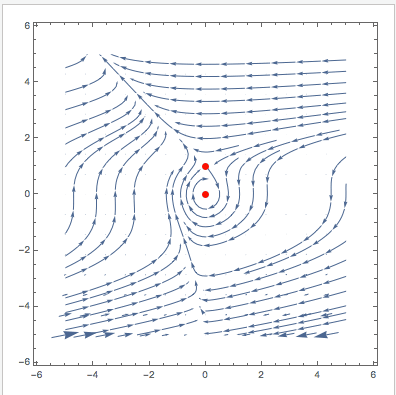
Cheers,

M.

PS: This modified version also plots the null-clines and indicates the stability of the fixed points.

Manipulate[
 colors = (Which[(Re[#[[1]]] == 0 && Re[#[[2]]] == 0), Blue, (Re[#[[1]]] < 0 && Re[#[[2]]] < 0), 
              Green, (Re[#[[1]]] > 0 && Re[#[[2]]] > 0), Red, True, Orange] & @
                Eigenvalues[D[{u (1 - u + (-1 + E^(-u \[Alpha])) v), v (\[Beta] - E^(-u \[Alpha]) \[Beta] - \[CapitalGamma])}, {{v, u}}] /. # /. {\[Alpha] -> \[Alpha]1, \[Beta] ->  \[Beta]1, \[CapitalGamma] -> \[CapitalGamma]1}]) & /@ (Select[ (sols \
/. {\[Alpha] -> \[Alpha]1, \[Beta] ->  \[Beta]1, \[CapitalGamma] -> \[CapitalGamma]1}), ! MemberQ[#[[All, 2]], Undefined] &]); 
Show[VectorPlot[{u (1 - u + (-1 + E^(-u \[Alpha]1)) v), v (\[Beta]1 - E^(-u \[Alpha]1) \[Beta]1 - \[CapitalGamma]1)}, {v, -5, 5}, {u, -5, 5}, StreamPoints -> 40, 
   Epilog -> Flatten@{PointSize[0.02], Red, {#[[1]], Point[#[[2]]]} & /@ Transpose[{colors, (Select[{v, u} /. (sols /. {\[Alpha] -> \[Alpha]1, \[Beta] ->  \[Beta]1, \[CapitalGamma] -> \[CapitalGamma]1}), !MemberQ[#, Undefined] &])}]}], ContourPlot[{u (1 - u + (-1 + E^(-u \[Alpha]1)) v) == 0, 
    v (\[Beta]1 - E^(-u \[Alpha]1) \[Beta]1 - \[CapitalGamma]1) == 0}, {v, -5, 5}, {u, -5, 5}, PlotPoints -> 30]], {{\[Alpha]1, 0.795}, 0, 2}, {{\[Beta]1, 1.79}, 0, 
  2}, {{\[CapitalGamma]1, 1.315}, 0, 3}]

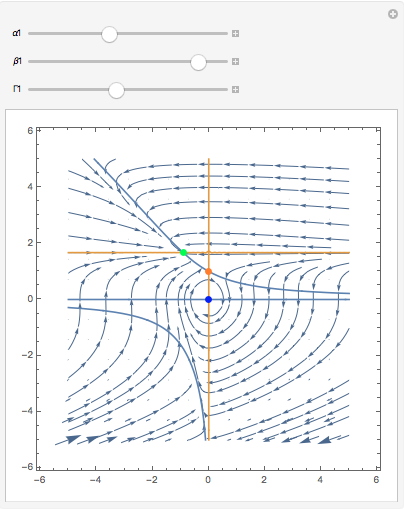
It is not very elegant in terms of the programming, but does the trick. Blue indicates non-hyperbolicity - so we cannot determine the stability using linearisation. Green is stable, orange is a saddle, and red would be unstable in both eigen-directions. Null-clines for the x-direction are in blue and for the y-direction in yellow/gold. Fixed points are where null-clines "of different colour" meet. You will see that in the code I calculate the Jacobian Matrix and evaluate at the fixed points. That means that the program calculates the eigenvalues, that - using the Hartman-Grobman theorem- determine the stability of hyperbolic fixed points.

I hope I haven't made a typo somewhere in the code...

Dear Murray,

you are quite right. My code was incomplete in the sense that it needed the lines from the original post, too. "sols" holds the solutions of the ODEs so that if you execute:

f[u, v] := u (1 - u + (-1 + E^(-u \[Alpha])) v);
g[u, v] := v (\[Beta] - E^(-u \[Alpha]) \[Beta] - \[CapitalGamma]);
sols = NSolve[{f[u, v] == 0, g[u, v] == 0}, {u, v}, Reals];

before the lines I posted, it should work. I noticed that in the original post the result of NSolve was saved in a variable equilibrio, which I renamed to sols. I certainly should have said that.

Sorry, Marco

PS: Note that the definition of the functions is taken from the original. I have not modified the definition of the function so something like:

f[u_, v_] := u (1 - u + (-1 + E^(-u \[Alpha])) v);
g[u_, v_] := v (\[Beta] - E^(-u \[Alpha]) \[Beta] - \[CapitalGamma]);
sols = NSolve[{f[u, v] == 0, g[u, v] == 0}, {u, v}, Reals];

which would look more like a standard definition.

Hi,

it appears that NSolve does a good job:

f[u, v] := u (1 - u + (-1 + E^(-u \[Alpha])) v);
g[u, v] := v (\[Beta] - E^(-u \[Alpha]) \[Beta] - \[CapitalGamma]);
NSolve[{f[u, v] == 0, g[u, v] == 0}, {u, v}, Reals]

gives

Cheers,

M.